π/2-θ、π/2+θの三角関数
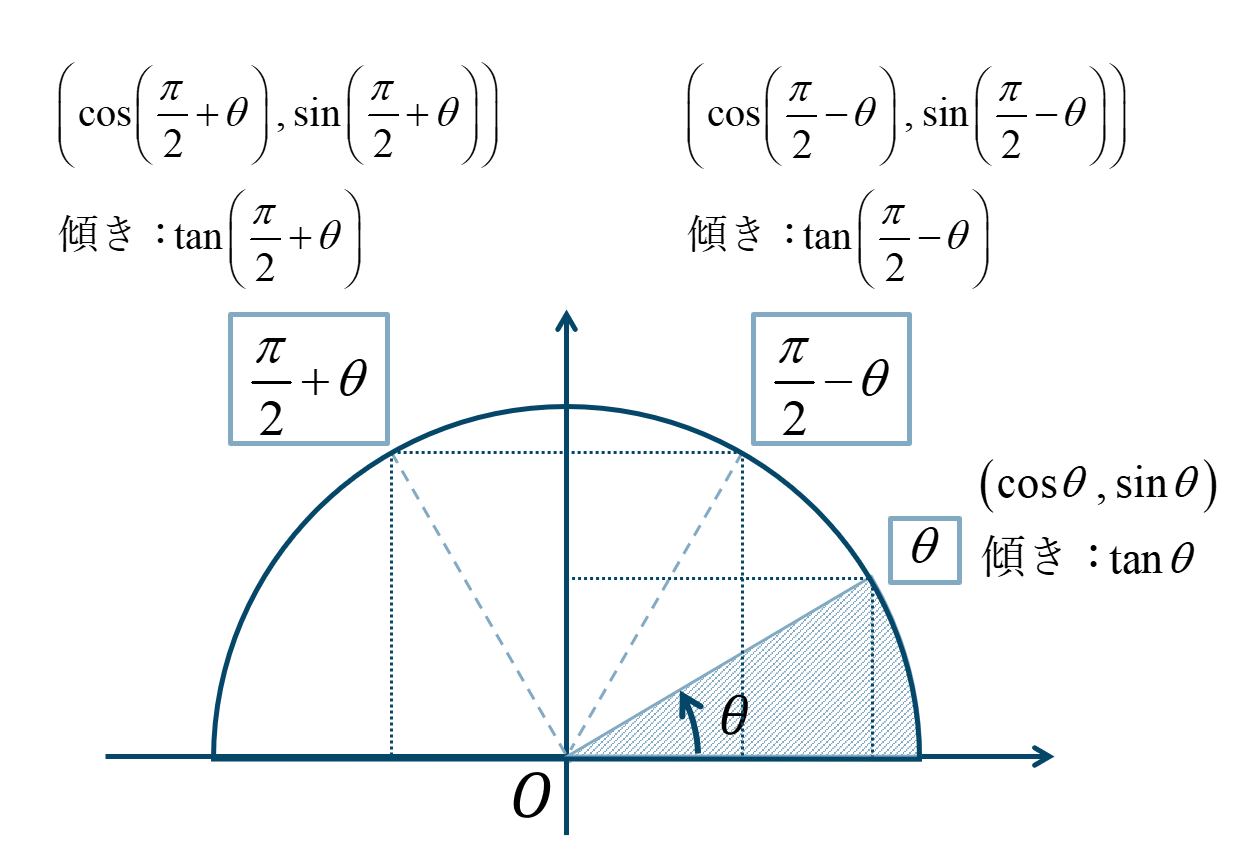
上の図の \(\theta\) の三角関数を基準として考えます。
① \({\Large \frac{\pi}{2}}-\theta\) の三角関数
\(y\) 座標は \(\theta\) のときの \(x\) 座標の値と同じになるので、
\(x\) 座標は \(\theta\) のときの \(y\) 座標の値と同じになるので、
傾きは \(\theta\) の傾きの逆数となるので、
② \({\Large \frac{\pi}{2}}+\theta\) の三角関数
\(y\) 座標は \(\theta\) のときの \(x\) 座標の値と同じになるので、
\(x\) 座標は \(\theta\) のときの \(y\) 座標と数値は同じで符号が逆になるので、
傾きは \(\theta\) の傾きの逆数で符号が逆になるので、
問題解説:三角関数の性質②
問題解説(1)
$$~~~~~~\sin{\left(\frac{\pi}{2}-\theta\right)}\cos{\theta}-\sin{\theta}\cos{\left(\frac{\pi}{2}+\theta\right)}$$それぞれの公式を用いて計算すると、$$~=\cos{\theta}\cos{\theta}-\sin{\theta}(-\sin{\theta})$$$$~=\cos^2{\theta}+\sin^2{\theta}$$$$~=1$$よって、答えは \(1\) となります。
問題解説(2)
$$~~~~~~\frac{\tan{\theta}\tan{\left({\Large \frac{\pi}{2}}-\theta\right)}\sin{\left({\Large \frac{\pi}{2}}+\theta\right)}}{\tan{\left({\Large \frac{\pi}{2}}+\theta\right)}\cos{\left({\Large \frac{\pi}{2}}-\theta\right)}}$$それぞれの公式を用いて計算すると、$$~=\frac{\tan{\theta}\cdot{\Large \frac{1}{\tan{\theta}}}\cdot\cos{\theta}}{\left(-{\Large \frac{1}{\tan{\theta}}}\right)\cdot\sin{\theta}}$$分母分子の \({\Large \frac{1}{\tan{\theta}}}\) で約分すると、$$~=-\frac{\tan{\theta}\cos{\theta}}{\sin{\theta}}$$次に、\(\tan{\theta}={\Large \frac{\sin{\theta}}{\cos{\theta}}}\) を用いると、$$~=-\frac{{\Large \frac{\sin{\theta}}{\cos{\theta}}}\cdot\cos{\theta}}{\sin{\theta}}$$$$~=-\frac{\sin{\theta}}{\sin{\theta}}$$$$~=-1$$よって、答えは\(-1\) となります。
今回のまとめ
余角の三角関数などの公式も単位円より、対応する値とその符号を確認して公式を導き出しましょう。



