数研出版：改訂版高等学校数学Ⅰ

p.124
練習1
\({\small (1)}~\sin{\theta}={\large \frac{2}{3}}~,~\cos{\theta}={\large \frac{\sqrt{5}}{3}}\)
　　\(\tan{\theta}={\large \frac{2}{\sqrt{5}}}\)
\({\small (2)}~\sin{\theta}={\large \frac{5}{13}}~,~\cos{\theta}={\large \frac{12}{13}}\)
　　\(\tan{\theta}={\large \frac{5}{12}}\)
\({\small (3)}~\sin{\theta}={\large \frac{\sqrt{7}}{4}}~,~\cos{\theta}={\large \frac{3}{4}}\)
　　\(\tan{\theta}={\large \frac{\sqrt{7}}{3}}\)
→ 直角三角形と三角比

p.124
練習2

	\(30^\circ\)	\(45^\circ\)	\(60^\circ\)
\(\sin{}\)	\({\large \frac{1}{2}}\)	\({\large \frac{1}{\sqrt{2}}}\)	\({\large \frac{\sqrt{3}}{2}}\)
\(\cos{}\)	\({\large \frac{\sqrt{3}}{2}}\)	\({\large \frac{1}{\sqrt{2}}}\)	\({\large \frac{1}{2}}\)
\(\tan{}\)	\({\large \frac{1}{\sqrt{3}}}\)	\(1\)	\(\sqrt{3}\)

→ 三角比の値（鋭角）

p.125
練習3
\({\small (1)}~0.2079\)　\({\small (2)}~0.6691\)
\({\small (3)}~3.7321\)

p.125
練習4
\({\small (1)}~24^\circ\)　\({\small (2)}~27^\circ\)

p.126
練習5
\(95\) m

p.127
練習6
\(32\) m

p.129
練習7
\(\sin{\theta}={\large \frac{2\sqrt{2}}{3}}~,~\tan{\theta}=2\sqrt{2}\)

p.129
練習8
\(\cos{\theta}={\large \frac{1}{\sqrt{3}}}~,~\sin{\theta}={\large \frac{\sqrt{6}}{3}}\)
→ 三角比の相互関係の公式（鋭角）

p.130
練習9
\({\small (1)}~28^\circ\)　\({\small (2)}~12^\circ\)　\({\small (3)}~67^\circ\)

p.130
練習10
\({\small (1)}~\cos{26^\circ}\)　\({\small (2)}~\sin{32^\circ}\)
\({\small (3)}~{\large \frac{1}{\tan{7^\circ}}}\)
→ 余角の公式

p.131
練習11
\({\small (1)}~r=\sqrt{2}~,~(-1,1)\)
　\(\sin{135^\circ}={\large \frac{1}{\sqrt{2}}}~,~\cos{135^\circ}=-{\large \frac{1}{\sqrt{2}}}\)
　\(\tan{135^\circ}=-1\)
\({\small (2)}~r=2~,~(-\sqrt{3},1)\)
　\(\sin{150^\circ}={\large \frac{1}{2}}~,~\cos{150^\circ}=-{\large \frac{\sqrt{3}}{2}}\)
　\(\tan{150^\circ}=-{\large \frac{1}{\sqrt{3}}}\)
→ 三角比の拡張

p.134
練習12
\({\small (1)}~0.6428\)　\({\small (2)}~-0.9135\)
\({\small (3)}~-5.6713\)
→ 補角の公式

p.135
練習13
\({\small (1)}~60^\circ~,~120^\circ\)　\({\small (2)}~120^\circ\)
\({\small (3)}~0^\circ~,~180^\circ\)

p.136
練習14
\({\small (1)}~45^\circ\)　\({\small (2)}~150^\circ\)
→ 三角比と方程式

p.137
練習15
\(\theta\) が鋭角のとき
　\(\cos{\theta}={\large \frac{3}{5}}~,~\tan{\theta}={\large \frac{4}{3}}\)
\(\theta\) が鈍角のとき
　\(\cos{\theta}=-{\large \frac{3}{5}}~,~\tan{\theta}=-{\large \frac{4}{3}}\)

p.137
練習16
\({\small (1)}~\sin{\theta}={\large \frac{2\sqrt{2}}{3}}~,~\tan{\theta}=-2\sqrt{2}\)
\({\small (2)}~\cos{\theta}=-{\large \frac{1}{\sqrt{5}}}~,~\sin{\theta}={\large \frac{2}{\sqrt{5}}}\)
→ 三角比の相互関係の公式（鈍角）

p.137
練習17
\({\small (1)}~45^\circ\)　\({\small (2)}~120^\circ\)
→ 直線の傾きと正接

p.139
1
\(18.4\) m

p.139
2
\({\small (1)}~16.2\)　\({\small (2)}~15.4\)

p.139
3
\({\small (1)}~\)[証明] \(\triangle {\rm ABC}\) の内角の和の条件より、
　\({\rm A+B+C}=180^\circ\)
よって、
　\({\rm B+C}=180^\circ-{\rm A}\)
これより、
　\({\large \frac{{\rm B+C}}{2}}=90^\circ-{\large \frac{{\rm A}}{2}}\)
ここで、\(\cos{(90^\circ-\theta)}=\sin{\theta}\) より、
　\(\cos{\left(90^\circ-{\large \frac{{\rm A}}{2}}\right)}=\sin{{\large \frac{{\rm A}}{2}}}\)
したがって、
　\(\sin{{\large \frac{{\rm A}}{2}}}=\cos{{\large \frac{{\rm B+C}}{2}}}\) [終]

\({\small (2)}~\)[証明] \(\triangle {\rm ABC}\) の内角の和の条件より、
　\({\rm A+B+C}=180^\circ\)
よって、
　\({\rm B+C}=180^\circ-{\rm A}\)
ここで、\(\sin{(180^\circ-\theta)}=\sin{\theta}\) より、
　\(\sin{(180^\circ-{\rm A})}=\sin{{\rm A}}\)
したがって、
　\(\sin{{\rm A}}=\sin{({\rm B+C})}\) [終]

p.139
4
\({\small (1)}~\)
　\(\cos{\theta}={\large \frac{\sqrt{7}}{4}}~,~\tan{\theta}={\large \frac{3}{\sqrt{7}}}\)
または
　\(\cos{\theta}=-{\large \frac{\sqrt{7}}{4}}~,~\tan{\theta}=-{\large \frac{3}{\sqrt{7}}}\)
\({\small (2)}~\)
　\(\sin{\theta}={\large \frac{3}{\sqrt{10}}}~,~\cos{\theta}=-{\large \frac{1}{\sqrt{10}}}\)

p.139
5
ア:\(1\)　イ:\(3\)　ウ:\(1\)　エ:\(0\)　オ:\(5\)

p.142
練習18
\({\small (1)}~{\large \frac{5\sqrt{2}}{2}}\)　\({\small (2)}~1\)

p.142
練習19
\(30^\circ~,~150^\circ\)

p.143
練習20
\({\small (1)}~b=1\)　\({\small (2)}~c=\sqrt{6}\)
→ 正弦定理

p.143
練習21
\(200\sqrt{2}\) m

p.144
練習22
[証明] \(\triangle {\rm CDB}\) において三平方の定理より、
　\({\rm BC}^2={\rm CD}^2+{\rm BD}^2\)
次に、\(\triangle {\rm CAD}\) において
　\(\sin{(180^\circ-{\rm A})}={\large \frac{{\rm CD}}{b}}\)
よって、
　\(b\sin{{\rm A}}={\rm CD}\)
２乗すると、
　\({\rm CD}^2=(b\sin{{\rm A}})^2\)
また、\(\triangle {\rm CAD}\) において、
　\(\cos{(180^\circ-{\rm A})}={\large \frac{{\rm AD}}{b}}\)
よって、
　\(-b\cos{{\rm A}}={\rm AD}\)
ここで、\({\rm BD=AB+AD}\) より、
　\({\rm BD}=c-b\cos{{\rm A}}\)
２乗すると、
　\({\rm BD}^2=(c-b\cos{{\rm A}})^2\) [終]

p.145
練習23
\({\small (1)}~b=\sqrt{5}\)　\({\small (2)}~c=7\)
→ 余弦定理

p.145
練習24
\(10\sqrt{21}\) m

p.146
練習25
\({\small (1)}~{\rm A}=30^\circ\)　\({\small (2)}~{\rm B}=135^\circ\)
→ 余弦定理

p.146
練習26
\({\small (1)}~\)鈍角　\({\small (2)}~\)鋭角
→ 三角形の辺と角の条件

p.147
練習27
\(b=2~,~{\rm A}=30^\circ~,~{\rm C}=105^\circ\)

p.148
練習28
\({\rm C}=120^\circ\)

p.150
練習29
\({\small (1)}~20\sqrt{2}\)　\({\small (2)}~{\large \frac{15}{2}}\)　\({\small (3)}~{\large \frac{\sqrt{3}}{4}}a^2\)

p.150
練習30
\({\small (1)}~10\sqrt{3}\)　\({\small (2)}~84\)
→ 三角形の面積（三角比）

p.151
練習31
\(6\sqrt{3}\)
→ 円に内接する四角形

p.152
練習32
\({\large \frac{\sqrt{15}}{6}}\)
→ 内接円の半径

p.153
発展1
\(14\sqrt{3}\)

p.154
練習33
\(50\sqrt{2}\) m

p.155
練習34
\(3\sqrt{29}\)
→ 直方体の計量

p.156
研究1
\({\large \frac{4\sqrt{11}}{3}}\)

p.157
6
\({\large \frac{3\sqrt{3}}{14}}\)

p.157
7
\({\small (1)}~{\large \frac{\sqrt{13}}{2}}\)　\({\small (2)}~{\large \frac{5}{2\sqrt{13}}}\)　\({\small (3)}~{\large \frac{\sqrt{37}}{2}}\)

p.157
8
\({\small (1)}~12\sqrt{3}\)　\({\small (2)}~12\)

p.157
9
\(3\sqrt{5}\)

p.157
10
ア:\(8\)　イ:\(3\)　ウ:\(2\)　エ:\(3\)　オ:\(3\)　カ:\(8\)　キ:\(3\)

p.158
1
\({\small (1)}~5(\sqrt{3}+1)\) m　\({\small (2)}~5(\sqrt{3}+3)\) m

p.158
2
\({\small (1)}~5\sqrt{3}\)　\({\small (2)}~5\sqrt{2}\)

p.158
3
[証明]

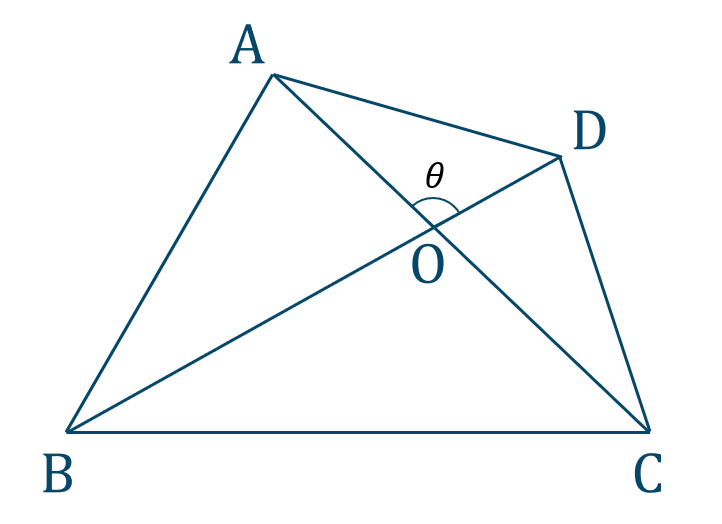
\(\triangle {\rm AOD}\) の面積は
　\({\large \frac{1}{2}}\cdot{\rm OA}\cdot{\rm OD}\sin{\theta}\)
\(\triangle {\rm AOB}\) の面積は
　　\({\large \frac{1}{2}}\cdot{\rm OA}\cdot{\rm OB}\sin{(180^\circ-\theta)}\)
　\(={\large \frac{1}{2}}\cdot{\rm OA}\cdot{\rm OB}\sin{\theta}\)
\(\triangle {\rm BOC}\) の面積は
　\({\large \frac{1}{2}}\cdot{\rm OB}\cdot{\rm OC}\sin{\theta}\)
\(\triangle {\rm OCD}\) の面積は
　　\({\large \frac{1}{2}}\cdot{\rm OC}\cdot{\rm OD}\sin{(180^\circ-\theta)}\)
　\(={\large \frac{1}{2}}\cdot{\rm OC}\cdot{\rm OD}\sin{\theta}\)
これら４つの三角形の面積の和が、四角形の面積 \(S\) となるので、
　\(S= {\large \frac{1}{2}}\cdot{\rm OA}\cdot{\rm OD}\sin{\theta}\)
　　　\(+ {\large \frac{1}{2}}\cdot{\rm OA}\cdot{\rm OB}\sin{\theta}\)
　　　\(~+{\large \frac{1}{2}}\cdot{\rm OB}\cdot{\rm OC}\sin{\theta}\)
　　　\(~~+ {\large \frac{1}{2}}\cdot{\rm OC}\cdot{\rm OD}\sin{\theta}\)
因数分解すると、
　\(S={\large \frac{1}{2}}({\rm OA+OC})({\rm OB+OD})\sin{\theta}\)
ここで、\({\rm OA+OC}=p~,~{\rm OB+OD}=q\) より、
　\(S={\large \frac{1}{2}}pq\sin{\theta}\) [終]

p.158
4
\({\small (1)}~\sqrt{3}+1\)　\({\small (2)}~{\large \frac{\sqrt{6}+\sqrt{2}}{4}}~,~{\large \frac{\sqrt{6}-\sqrt{2}}{4}}\)

p.159
5
\(a=4~,~{\rm A}=90^\circ~,~{\rm B}=60^\circ\)
または
\(a=2~,~{\rm A}=30^\circ~,~{\rm B}=120^\circ\)

p.158
6
\({\large \frac{7\sqrt{55}}{4}}\)

p.158
7
\({\small (1)}~14\)　\({\small (2)}~{\large \frac{12}{7}}\)

p.158
8
\({\small (1)}~{\large \frac{\sqrt{2}}{12}}\)　\({\small (2)}~{\large \frac{\sqrt{2}}{48}}\)　\({\small (3)}~{\large \frac{\sqrt{6}}{12}}\)