文字数が多く、重くなるのでページを分割しています。
各章は下のリンクまたはページ下の「次へ」をクリックしてください。
第1章 数と式
第2章 集合と命題
第4章 図形と計量
第5章 データの分析
第3章 2次関数
第1節 2次関数とグラフ
p.68
練習1
\({\small (1)}~5\) \({\small (2)}~-1\) \({\small (3)}~8\) \({\small (4)}~-3a+2\)
練習1
\({\small (1)}~5\) \({\small (2)}~-1\) \({\small (3)}~8\) \({\small (4)}~-3a+2\)
p.70
問3
\({\small (1)}~\)第1象限 \({\small (2)}~\)第4象限
\({\small (3)}~\)第3象限 \({\small (4)}~\)第2象限
→ 関数の値と象限
問3
\({\small (1)}~\)第1象限 \({\small (2)}~\)第4象限
\({\small (3)}~\)第3象限 \({\small (4)}~\)第2象限
→ 関数の値と象限
p.71
練習3
\({\small (1)}~\)

\({\small (2)}~\)

練習3
\({\small (1)}~\)
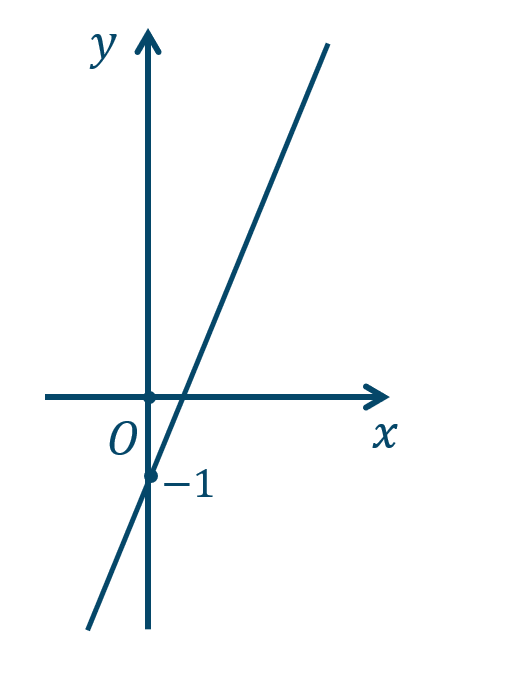
\({\small (2)}~\)

p.71
問1

\(-1≦y<7\)
問1
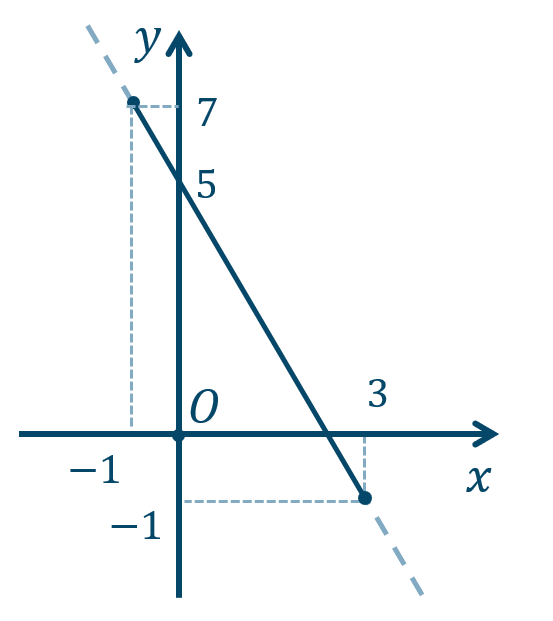
\(-1≦y<7\)
p.72
練習5
\({\small (1)}~\)
\(x=2\) で、最大値 \(3\)
\(x=-4\) で、最大値 \(0\)
\({\small (2)}~\)
\(x=-3\) で、最大値 \(-2\)
\(x=1\) で、最大値 \(-6\)
\({\small (3)}~\)
\(x=0\) で、最大値 \(5\)
最小値なし
\({\small (4)}~\)
最大値なし
最小値なし
→ 関数の値域と最大値・最小値
練習5
\({\small (1)}~\)
\(x=2\) で、最大値 \(3\)
\(x=-4\) で、最大値 \(0\)
\({\small (2)}~\)
\(x=-3\) で、最大値 \(-2\)
\(x=1\) で、最大値 \(-6\)
\({\small (3)}~\)
\(x=0\) で、最大値 \(5\)
最小値なし
\({\small (4)}~\)
最大値なし
最小値なし
→ 関数の値域と最大値・最小値
p.75
練習7
\({\small (1)}~(-2,4)\) \({\small (2)}~(1,1)\)
\({\small (3)}~(-5,3)\) \({\small (4)}~(-4,-1)\)
練習7
\({\small (1)}~(-2,4)\) \({\small (2)}~(1,1)\)
\({\small (3)}~(-5,3)\) \({\small (4)}~(-4,-1)\)
p.76
問2

問2
p.76
練習8
\({\small (1)}~\)

軸は \(y\) 軸、頂点 \((0,1)\)
\({\small (2)}~\)

軸は \(y\) 軸、頂点 \((0,-3)\)
\({\small (3)}~\)

軸は \(y\) 軸、頂点 \((0,2)\)
練習8
\({\small (1)}~\)
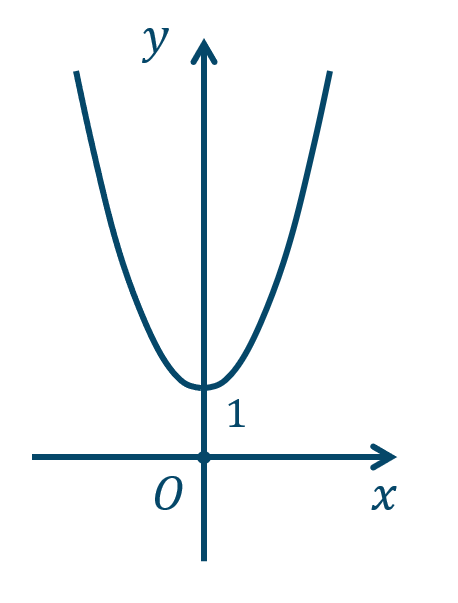
軸は \(y\) 軸、頂点 \((0,1)\)
\({\small (2)}~\)
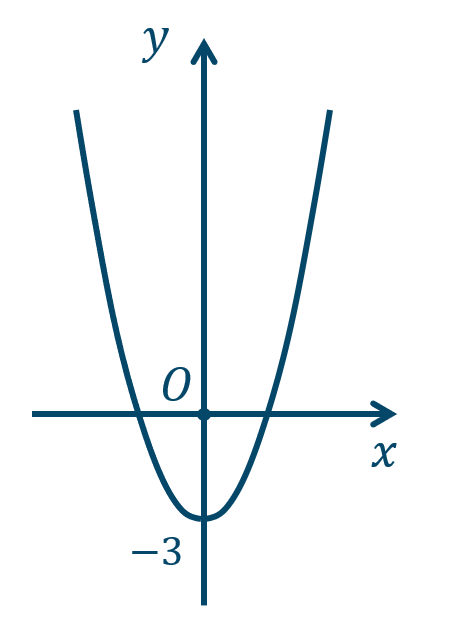
軸は \(y\) 軸、頂点 \((0,-3)\)
\({\small (3)}~\)
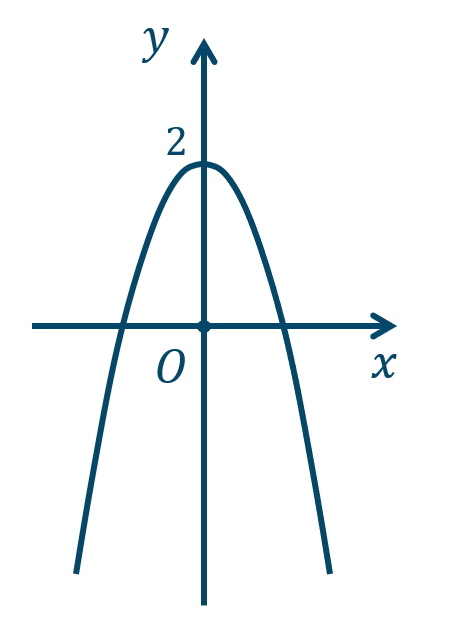
軸は \(y\) 軸、頂点 \((0,2)\)
p.77
問3

問3

p.77
練習9
\({\small (1)}~\)

軸は \(x=1\)、頂点 \((1,0)\)
\({\small (2)}~\)

軸は \(x=-2\)、頂点 \((-2,0)\)
\({\small (3)}~\)

軸は \(x=-3\)、頂点 \((-3,0)\)
練習9
\({\small (1)}~\)
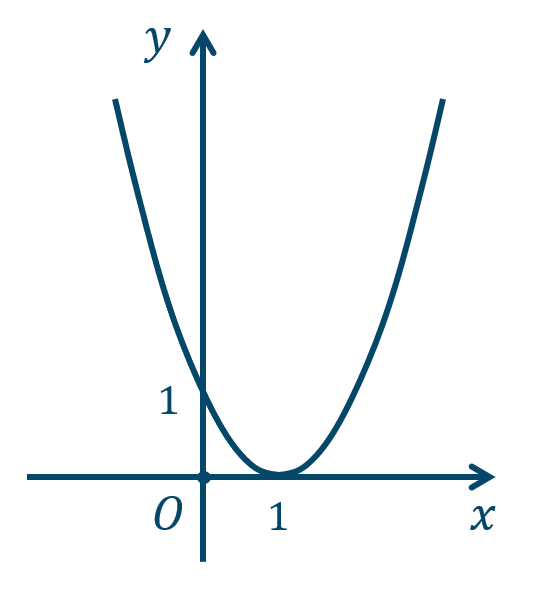
軸は \(x=1\)、頂点 \((1,0)\)
\({\small (2)}~\)
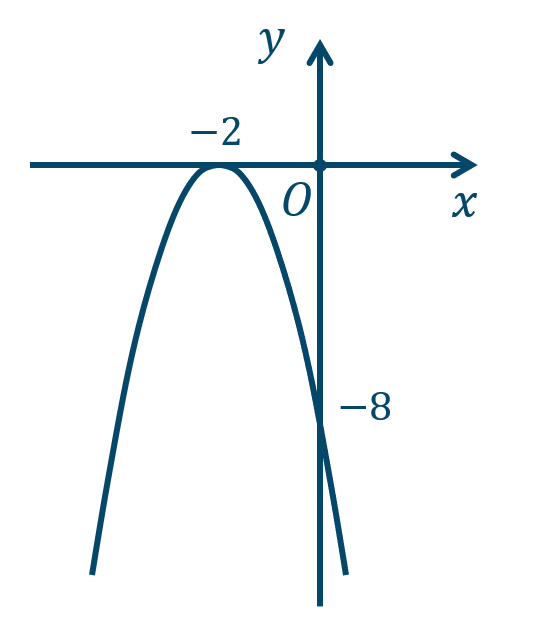
軸は \(x=-2\)、頂点 \((-2,0)\)
\({\small (3)}~\)
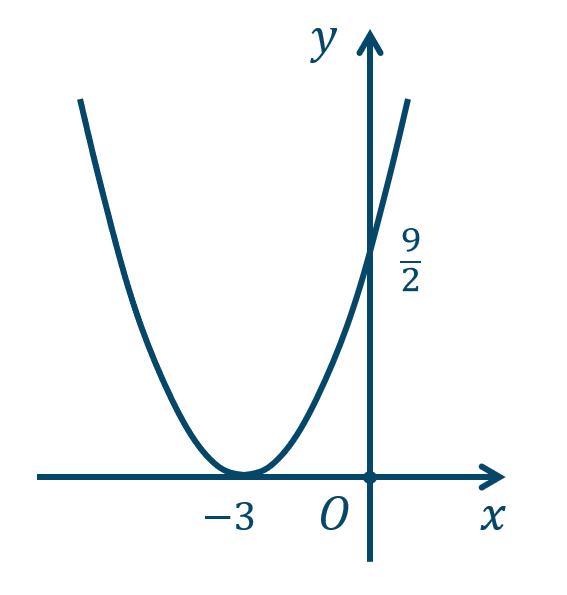
軸は \(x=-3\)、頂点 \((-3,0)\)
p.78
練習10
\({\small (1)}~\)

軸は \(x=1\)、頂点 \((1,2)\)
\({\small (2)}~\)

軸は \(x=2\)、頂点 \((2,-3)\)
\({\small (3)}~\)

軸は \(x=-1\)、頂点 \((-1,-2)\)
\({\small (4)}~\)

軸は \(x=-2\)、頂点 \((-2,5)\)
→ 2次関数のグラフ
練習10
\({\small (1)}~\)
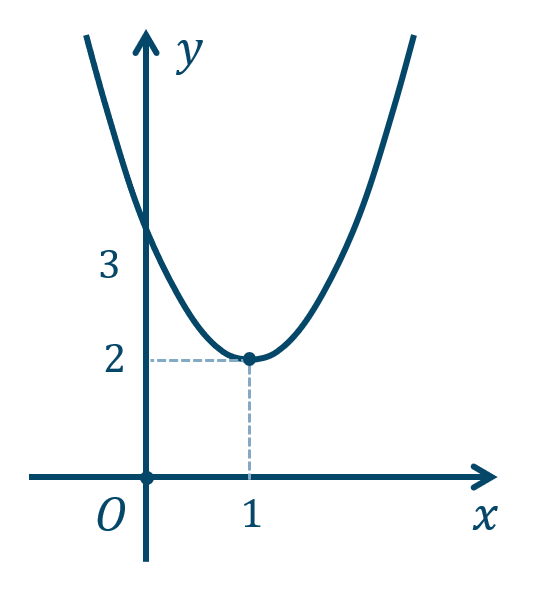
軸は \(x=1\)、頂点 \((1,2)\)
\({\small (2)}~\)
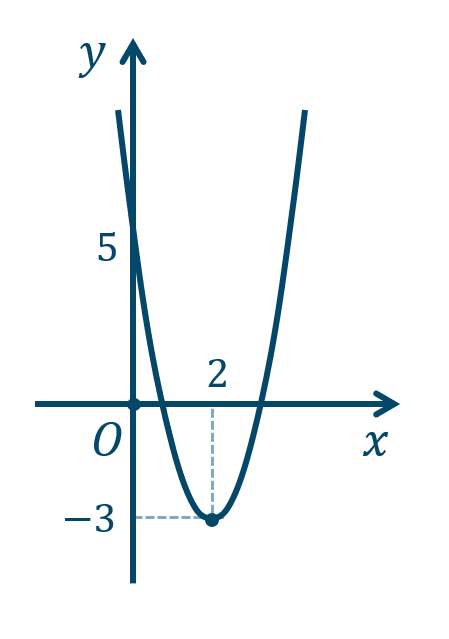
軸は \(x=2\)、頂点 \((2,-3)\)
\({\small (3)}~\)
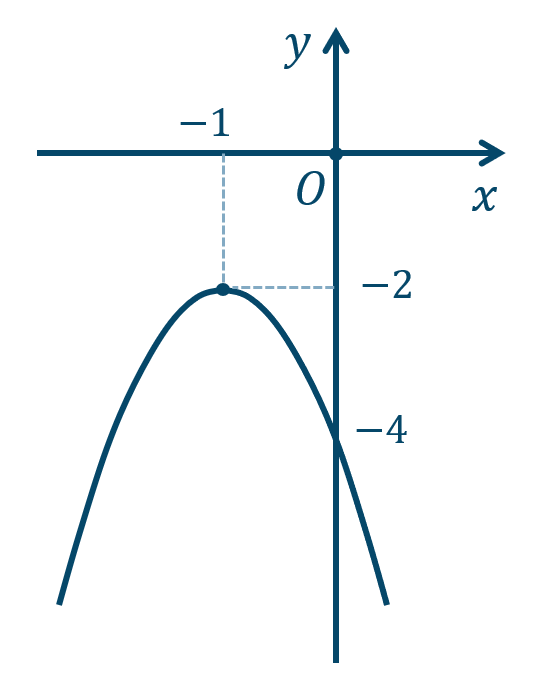
軸は \(x=-1\)、頂点 \((-1,-2)\)
\({\small (4)}~\)
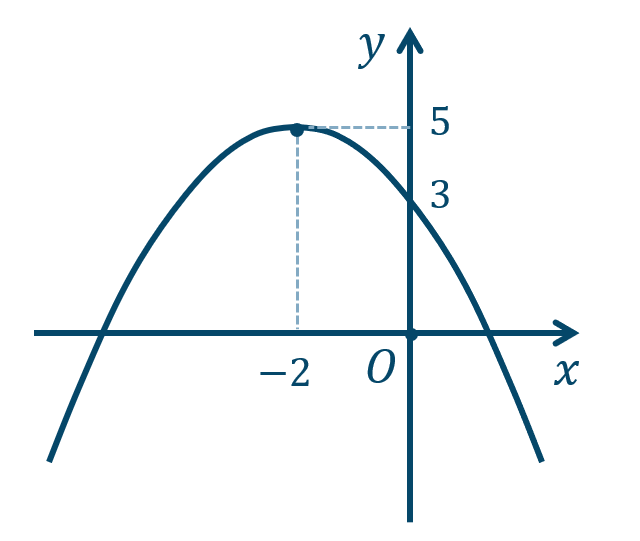
軸は \(x=-2\)、頂点 \((-2,5)\)
→ 2次関数のグラフ
p.79
練習11
\({\small (1)}~(x-2)^2+1\)
\({\small (2)}~2(x+2)^2-1\)
\({\small (3)}~\left(x-{\large \frac{1}{2}}\right)^2-{\large \frac{9}{4}}\)
\({\small (4)}~2\left(x+{\large \frac{3}{2}}\right)^2-{\large \frac{11}{2}}\)
→ 2次関数の平方完成
練習11
\({\small (1)}~(x-2)^2+1\)
\({\small (2)}~2(x+2)^2-1\)
\({\small (3)}~\left(x-{\large \frac{1}{2}}\right)^2-{\large \frac{9}{4}}\)
\({\small (4)}~2\left(x+{\large \frac{3}{2}}\right)^2-{\large \frac{11}{2}}\)
→ 2次関数の平方完成
p.78
練習12
\({\small (1)}~\)

軸は \(x=3\)、頂点 \((3,-5)\)
\({\small (2)}~\)

軸は \(x=-1\)、頂点 \((-1,1)\)
\({\small (3)}~\)

軸は \(x=2\)、頂点 \((2,4)\)
\({\small (4)}~\)

軸は \(x=-1\)、頂点 \((-1,-2)\)
\({\small (5)}~\)

軸は \(x={\large \frac{1}{2}}\)、頂点 \(\left({\large \frac{1}{2}},{\large \frac{3}{2}}\right)\)
\({\small (6)}~\)

軸は \(x=-3\)、頂点 \(\left(-3,{\large \frac{9}{2}}\right)\)
→ 2次関数の平方完成
練習12
\({\small (1)}~\)
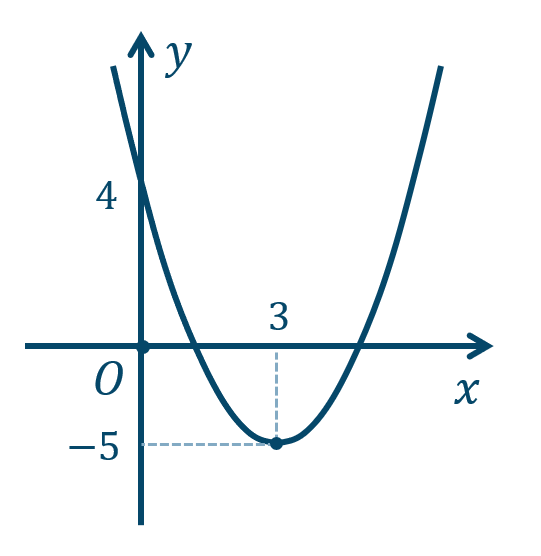
軸は \(x=3\)、頂点 \((3,-5)\)
\({\small (2)}~\)
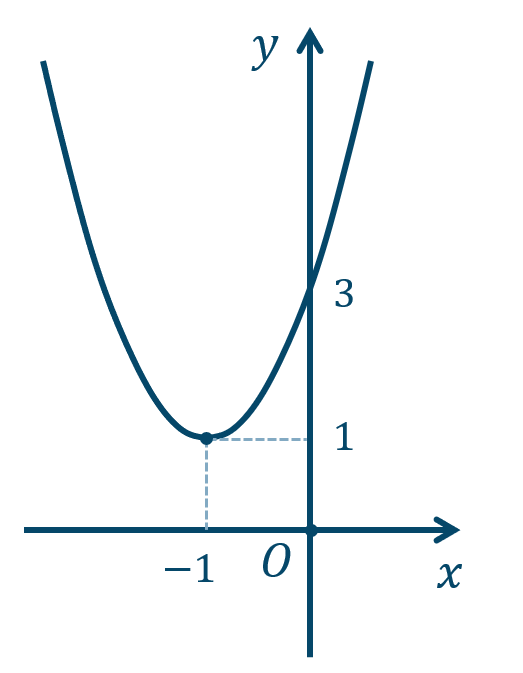
軸は \(x=-1\)、頂点 \((-1,1)\)
\({\small (3)}~\)
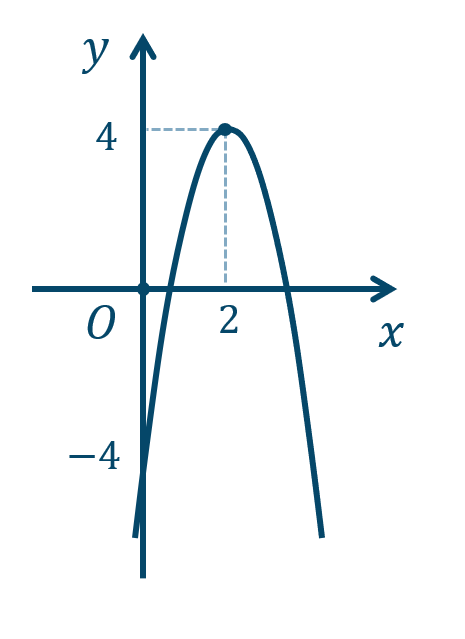
軸は \(x=2\)、頂点 \((2,4)\)
\({\small (4)}~\)
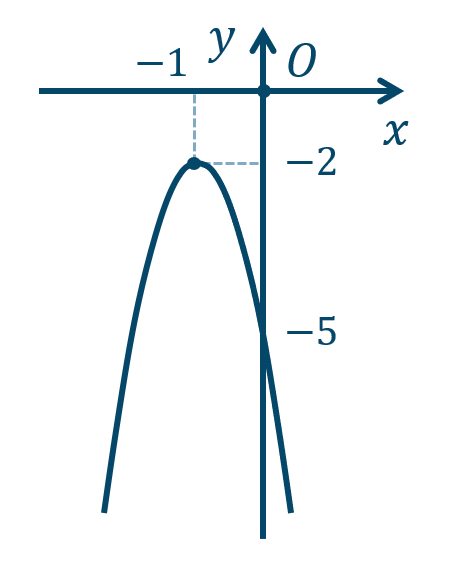
軸は \(x=-1\)、頂点 \((-1,-2)\)
\({\small (5)}~\)
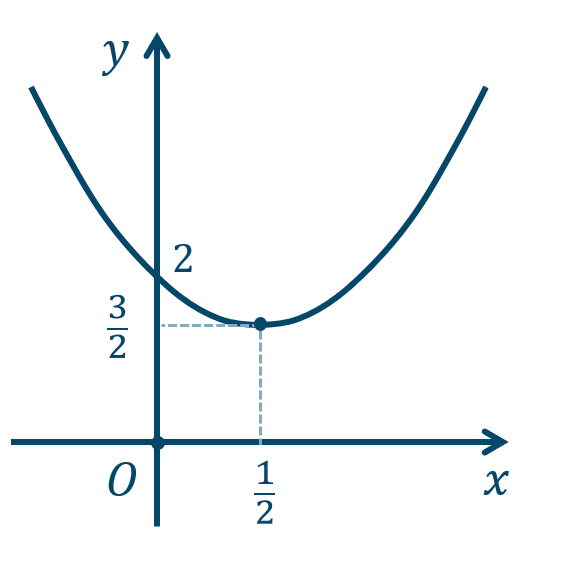
軸は \(x={\large \frac{1}{2}}\)、頂点 \(\left({\large \frac{1}{2}},{\large \frac{3}{2}}\right)\)
\({\small (6)}~\)
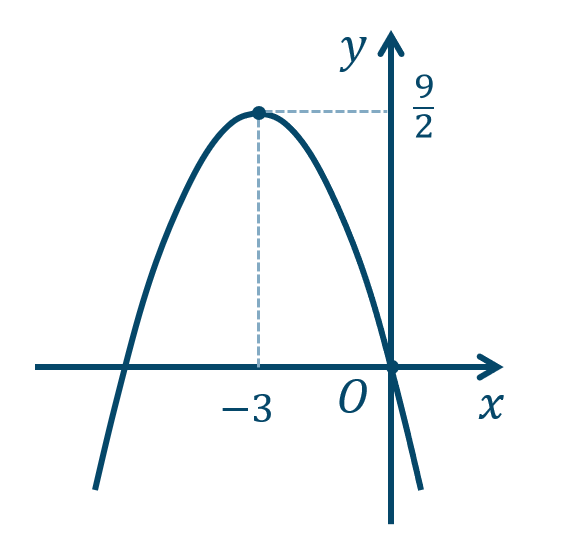
軸は \(x=-3\)、頂点 \(\left(-3,{\large \frac{9}{2}}\right)\)
→ 2次関数の平方完成
p.83
練習14
\({\small (1)}~y=3x^2+18x+29\)
\({\small (2)}~y=-2x^2-12x-15\)
\({\small (3)}~y=x^2+9x+16\)
→ 平行移動後のグラフ
練習14
\({\small (1)}~y=3x^2+18x+29\)
\({\small (2)}~y=-2x^2-12x-15\)
\({\small (3)}~y=x^2+9x+16\)
→ 平行移動後のグラフ
p.84
練習15
\({\small (1)}~y=-2x^2+4x-5\)
\({\small (2)}~y=2x^2+4x+5\)
\({\small (3)}~y=-2x^2-4x-5\)
→ グラフの対称移動
練習15
\({\small (1)}~y=-2x^2+4x-5\)
\({\small (2)}~y=2x^2+4x+5\)
\({\small (3)}~y=-2x^2-4x-5\)
→ グラフの対称移動
p.87
練習16
\({\small (1)}~\)
最大値なし
最小値 \(-2~(x=-2)\)
\({\small (2)}~\)
最大値 \(5~(x=3)\)
最小値なし
\({\small (3)}~\)
最大値なし
最小値 \(1~(x=-1)\)
\({\small (2)}~\)
最大値 \({\large \frac{9}{2}}~\left(x=-{\large \frac{3}{2}}\right)\)
最小値なし
練習16
\({\small (1)}~\)
最大値なし
最小値 \(-2~(x=-2)\)
\({\small (2)}~\)
最大値 \(5~(x=3)\)
最小値なし
\({\small (3)}~\)
最大値なし
最小値 \(1~(x=-1)\)
\({\small (2)}~\)
最大値 \({\large \frac{9}{2}}~\left(x=-{\large \frac{3}{2}}\right)\)
最小値なし
p.87
練習17
\({\small (1)}~\)
最大値 \(0~(x=1)\)
最小値 \(-8~(x=3)\)
\({\small (2)}~\)
最大値 \(7~(x=-1)\)
最小値 \(-1~(x=1)\)
練習17
\({\small (1)}~\)
最大値 \(0~(x=1)\)
最小値 \(-8~(x=3)\)
\({\small (2)}~\)
最大値 \(7~(x=-1)\)
最小値 \(-1~(x=1)\)
p.88
練習18
\({\small (1)}~\)
最大値なし
最小値 \(-1~(x=-1)\)
\({\small (2)}~\)
最大値 \({\large \frac{17}{8}}~\left(x={\large \frac{3}{4}}\right)\)
最小値 \(-1~(x=2)\)
→ 2次関数の最大値・最小値
練習18
\({\small (1)}~\)
最大値なし
最小値 \(-1~(x=-1)\)
\({\small (2)}~\)
最大値 \({\large \frac{17}{8}}~\left(x={\large \frac{3}{4}}\right)\)
最小値 \(-1~(x=2)\)
→ 2次関数の最大値・最小値
p.89
練習20
\(0<a<1\) のとき
\(x=a\) で最大値 \(-a^2+2a+1\)
\(1≦a\) のとき
\(x=1\) で最大値 \(2\)
→ 定義域が変化する2次関数の最大値・最小値
練習20
\(0<a<1\) のとき
\(x=a\) で最大値 \(-a^2+2a+1\)
\(1≦a\) のとき
\(x=1\) で最大値 \(2\)
→ 定義域が変化する2次関数の最大値・最小値
p.89
問4
\({\small (1)}~a=4\)
\({\small (2)}~\)
\(0<a<4\) のとき
\(x=0\) で最小値 \(1\)
\(a=4\) のとき
\(x=0~,~4\) で最小値 \(1\)
\(4<a\) のとき
\(x=a\) で最小値 \(a^2-4a+1\)
問4
\({\small (1)}~a=4\)
\({\small (2)}~\)
\(0<a<4\) のとき
\(x=0\) で最小値 \(1\)
\(a=4\) のとき
\(x=0~,~4\) で最小値 \(1\)
\(4<a\) のとき
\(x=a\) で最小値 \(a^2-4a+1\)
p.90
練習21
\(a<0\) のとき
\(x=0\) で最小値 \(2a^2\)
\(0≦a≦1\) のとき
\(x=a\) で最小値 \(0\)
\(1<a\) のとき
\(x=1\) で最小値 \(2a^2-4a+2\)
→ 文字係数を含む2次関数の最大値・最小値
練習21
\(a<0\) のとき
\(x=0\) で最小値 \(2a^2\)
\(0≦a≦1\) のとき
\(x=a\) で最小値 \(0\)
\(1<a\) のとき
\(x=1\) で最小値 \(2a^2-4a+2\)
→ 文字係数を含む2次関数の最大値・最小値
p.90
問5
\(a<1\) のとき
\(x=2\) で最大値 \(a^2-4a+5\)
\(a=1\) のとき
\(x=0,2\) で最大値 \(2\)
\(1<a\) のとき
\(x=0\) で最大値 \(a^2+1\)
→ 文字係数を含む2次関数の最大値・最小値
問5
\(a<1\) のとき
\(x=2\) で最大値 \(a^2-4a+5\)
\(a=1\) のとき
\(x=0,2\) で最大値 \(2\)
\(1<a\) のとき
\(x=0\) で最大値 \(a^2+1\)
→ 文字係数を含む2次関数の最大値・最小値
p.94
練習24
\({\small (1)}~y=-x^2+4x-1\)
\({\small (2)}~y={\large \frac{1}{4}}x^2+x-4\)
→ 2次関数の決定①(頂点)
練習24
\({\small (1)}~y=-x^2+4x-1\)
\({\small (2)}~y={\large \frac{1}{4}}x^2+x-4\)
→ 2次関数の決定①(頂点)
p.96
練習25
\({\small (1)}~a=-1~,~b=4~,~c=6\)
\({\small (2)}~x=2~,~y=-2~,~z=1\)
練習25
\({\small (1)}~a=-1~,~b=4~,~c=6\)
\({\small (2)}~x=2~,~y=-2~,~z=1\)
問題
p.97
1
\({\small (1)}~\)

\(-6<y≦{\large \frac{1}{4}}\)
\({\small (2)}~\)

\(-6≦y≦{\large \frac{1}{8}}\)
\({\small (3)}~\)

\(-4≦y≦5\)
1
\({\small (1)}~\)

\(-6<y≦{\large \frac{1}{4}}\)
\({\small (2)}~\)
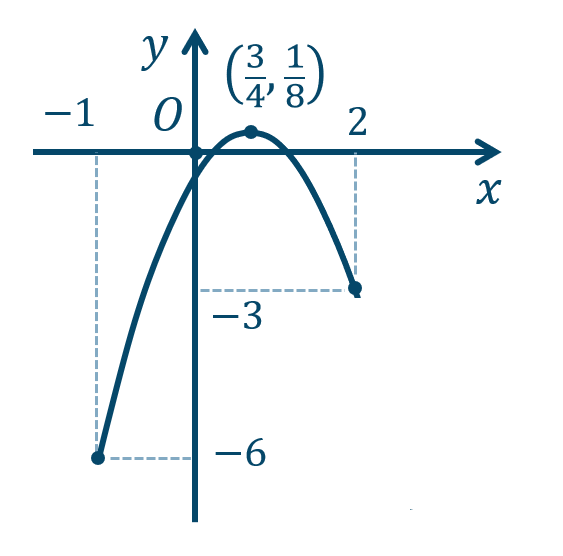
\(-6≦y≦{\large \frac{1}{8}}\)
\({\small (3)}~\)
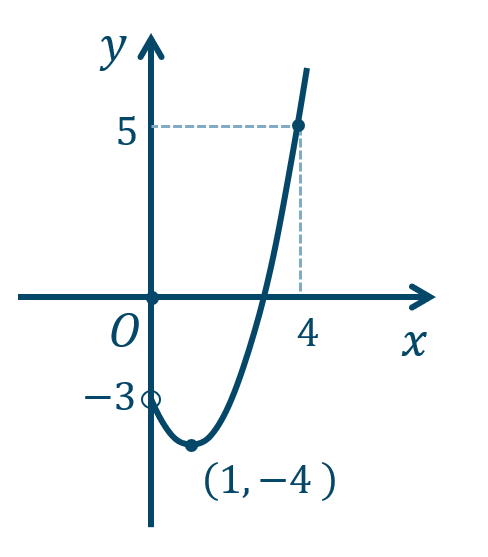
\(-4≦y≦5\)
p.97
2
\({\small (1)}~3\) 秒後、\(45\) m
\({\small (2)}~6\) 秒後
2
\({\small (1)}~3\) 秒後、\(45\) m
\({\small (2)}~6\) 秒後
p.97
3
\(2\)
3
\(2\)
p.97
4
\({\small (1)}~\)
\(a<0\) のとき
\(x=0\) で最大値 \(1\)
\(0≦x≦2\) のとき
\(x=a\) で最大値 \(a^2+1\)
\(2<a\) のとき
\(x=2\) で最大値 \(4a-3\)
\({\small (2)}~\)
\(a<1\) のとき
\(x=2\) で最小値 \(4a-3\)
\(a=1\) のとき
\(x=0,2\) で最小値 \(1\)
\(1<a\) のとき
\(x=0\) で最小値 \(1\)
4
\({\small (1)}~\)
\(a<0\) のとき
\(x=0\) で最大値 \(1\)
\(0≦x≦2\) のとき
\(x=a\) で最大値 \(a^2+1\)
\(2<a\) のとき
\(x=2\) で最大値 \(4a-3\)
\({\small (2)}~\)
\(a<1\) のとき
\(x=2\) で最小値 \(4a-3\)
\(a=1\) のとき
\(x=0,2\) で最小値 \(1\)
\(1<a\) のとき
\(x=0\) で最小値 \(1\)
p.97
5
\({\small (1)}~y=(x-3)^2-5\)
\({\small (2)}~y=2x^2-4x-6\)
5
\({\small (1)}~y=(x-3)^2-5\)
\({\small (2)}~y=2x^2-4x-6\)
p.97
6
\(y=-(x-2)^2+8\)
6
\(y=-(x-2)^2+8\)
第2節 2次方程式と2次不等式
p.98
練習27
\({\small (1)}~x=1~,~5\)
\({\small (2)}~x=8~,~-3\)
\({\small (3)}~x=-2~,~-{\large \frac{1}{2}}\)
\({\small (4)}~x=-3~,~{\large \frac{2}{3}}\)
練習27
\({\small (1)}~x=1~,~5\)
\({\small (2)}~x=8~,~-3\)
\({\small (3)}~x=-2~,~-{\large \frac{1}{2}}\)
\({\small (4)}~x=-3~,~{\large \frac{2}{3}}\)
p.100
練習28
\({\small (1)}~x={\large \frac{-7\pm\sqrt{37}}{6}}\)
\({\small (2)}~x={\large \frac{3\pm\sqrt{17}}{2}}\)
\({\small (3)}~x=-1\pm\sqrt{2}\)
\({\small (4)}~x={\large \frac{2\pm3\sqrt{2}}{2}}\)
\({\small (5)}~x={\large \frac{2}{3}}\)
\({\small (6)}~x=\sqrt{3}\pm1\)
→ 2次方程式の解
練習28
\({\small (1)}~x={\large \frac{-7\pm\sqrt{37}}{6}}\)
\({\small (2)}~x={\large \frac{3\pm\sqrt{17}}{2}}\)
\({\small (3)}~x=-1\pm\sqrt{2}\)
\({\small (4)}~x={\large \frac{2\pm3\sqrt{2}}{2}}\)
\({\small (5)}~x={\large \frac{2}{3}}\)
\({\small (6)}~x=\sqrt{3}\pm1\)
→ 2次方程式の解
p.102
問6
\(x=\sqrt{2}~,~x=-\sqrt{2}\)
問6
\(x=\sqrt{2}~,~x=-\sqrt{2}\)
p.103
練習32
\({\small (1)}~\left({\large \frac{-2+\sqrt{6}}{2}},0\right)~,~\left({\large \frac{-2-\sqrt{6}}{2}},0\right)\)
\({\small (2)}~\left({\large \frac{3}{2}},0\right)\)
→ 2次関数とx軸との交点
練習32
\({\small (1)}~\left({\large \frac{-2+\sqrt{6}}{2}},0\right)~,~\left({\large \frac{-2-\sqrt{6}}{2}},0\right)\)
\({\small (2)}~\left({\large \frac{3}{2}},0\right)\)
→ 2次関数とx軸との交点
p.106
練習33
\({\small (1)}~\)2個 \({\small (2)}~\)0個 \({\small (3)}~\)1個
練習33
\({\small (1)}~\)2個 \({\small (2)}~\)0個 \({\small (3)}~\)1個
p.106
練習34
\(m<{\large \frac{1}{4}}\) のとき 2個
\(m={\large \frac{1}{4}}\) のとき 1個
\(m>{\large \frac{1}{4}}\) のとき 0個
→ 2次関数とx軸との交点の条件
練習34
\(m<{\large \frac{1}{4}}\) のとき 2個
\(m={\large \frac{1}{4}}\) のとき 1個
\(m>{\large \frac{1}{4}}\) のとき 0個
→ 2次関数とx軸との交点の条件
p.108
発展1
\({\small (1)}~(2,-3)~,~(7,-18)\)
\({\small (2)}~(-2,9)\)
発展1
\({\small (1)}~(2,-3)~,~(7,-18)\)
\({\small (2)}~(-2,9)\)
p.109
練習35
\({\small (1)}~x>1\) \({\small (2)}~x>{\large \frac{5}{3}}\)
\({\small (3)}~x≦-{\large \frac{2}{3}}\) \({\small (4)}~x≦-{\large \frac{1}{2}}\)
練習35
\({\small (1)}~x>1\) \({\small (2)}~x>{\large \frac{5}{3}}\)
\({\small (3)}~x≦-{\large \frac{2}{3}}\) \({\small (4)}~x≦-{\large \frac{1}{2}}\)
p.111
練習36
\({\small (1)}~x<3~,~5<x\)
\({\small (2)}~-3≦x≦2\)
\({\small (3)}~3<x<4\)
\({\small (4)}~x≦-4~,~1≦x\)
\({\small (5)}~-3≦x≦-2\)
\({\small (6)}~x≦-2~,~2≦x\)
→ 2次不等式の解①(因数分解)
練習36
\({\small (1)}~x<3~,~5<x\)
\({\small (2)}~-3≦x≦2\)
\({\small (3)}~3<x<4\)
\({\small (4)}~x≦-4~,~1≦x\)
\({\small (5)}~-3≦x≦-2\)
\({\small (6)}~x≦-2~,~2≦x\)
→ 2次不等式の解①(因数分解)
p.112
練習37
\({\small (1)}~{\large \frac{1}{2}}<x<3\)
\({\small (2)}~x≦-{\large \frac{1}{2}}~,~2≦x\)
\({\small (3)}~-1-\sqrt{2}≦x≦-1+\sqrt{2}\)
\({\small (4)}~x<{\large \frac{-1-\sqrt{13}}{6}}~,~{\large \frac{-1+\sqrt{13}}{6}}<x\)
→ 2次不等式の解③(解の公式)
練習37
\({\small (1)}~{\large \frac{1}{2}}<x<3\)
\({\small (2)}~x≦-{\large \frac{1}{2}}~,~2≦x\)
\({\small (3)}~-1-\sqrt{2}≦x≦-1+\sqrt{2}\)
\({\small (4)}~x<{\large \frac{-1-\sqrt{13}}{6}}~,~{\large \frac{-1+\sqrt{13}}{6}}<x\)
→ 2次不等式の解③(解の公式)
p.112
問7
\(x<1~,~2<x\)
問7
\(x<1~,~2<x\)
p.112
練習38
\({\small (1)}~{\large \frac{3-\sqrt{13}}{2}}<x<{\large \frac{3+\sqrt{13}}{2}}\)
\({\small (2)}~x≦{\large \frac{3-\sqrt{7}}{2}}~,~{\large \frac{3+\sqrt{7}}{2}}≦x\)
→ 2次不等式の解③(解の公式)
練習38
\({\small (1)}~{\large \frac{3-\sqrt{13}}{2}}<x<{\large \frac{3+\sqrt{13}}{2}}\)
\({\small (2)}~x≦{\large \frac{3-\sqrt{7}}{2}}~,~{\large \frac{3+\sqrt{7}}{2}}≦x\)
→ 2次不等式の解③(解の公式)
p.113
練習39
\({\small (1)}~x=-3\) 以外のすべての実数
\({\small (2)}~\)すべての実数
\({\small (3)}~\)解なし
\({\small (4)}~x=\sqrt{3}\)
→ 2次不等式の解②(x軸と接する)
練習39
\({\small (1)}~x=-3\) 以外のすべての実数
\({\small (2)}~\)すべての実数
\({\small (3)}~\)解なし
\({\small (4)}~x=\sqrt{3}\)
→ 2次不等式の解②(x軸と接する)
p.114
練習40
\({\small (1)}~\)すべての実数
\({\small (2)}~\)すべての実数
\({\small (3)}~\)解なし
\({\small (4)}~\)解なし
→ 2次不等式の解④(交点がない)
練習40
\({\small (1)}~\)すべての実数
\({\small (2)}~\)すべての実数
\({\small (3)}~\)解なし
\({\small (4)}~\)解なし
→ 2次不等式の解④(交点がない)
p.117
練習44
\({\small (1)}~-1<x≦3\)
\({\small (2)}~-{\large \frac{5}{3}}<x<-1~,~{\large \frac{3}{2}}<x<2\)
→ 連立2次不等式の解
練習44
\({\small (1)}~-1<x≦3\)
\({\small (2)}~-{\large \frac{5}{3}}<x<-1~,~{\large \frac{3}{2}}<x<2\)
→ 連立2次不等式の解
p.117
問8
\(-4≦x<-3~,~1<x≦2\)
問8
\(-4≦x<-3~,~1<x≦2\)
p.118
練習46
\(1≦t≦3~,~7≦t≦9\)
練習46
\(1≦t≦3~,~7≦t≦9\)
p.120
研究1
\({\small (1)}~\)

\({\small (2)}~\)

\({\small (3)}~\)

研究1
\({\small (1)}~\)
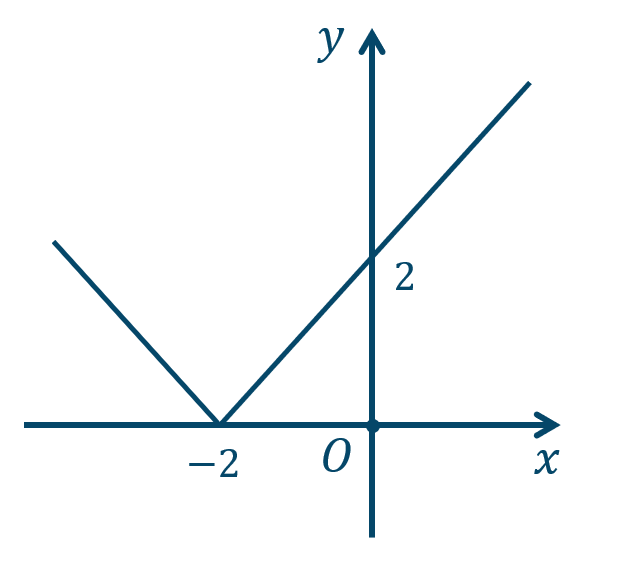
\({\small (2)}~\)
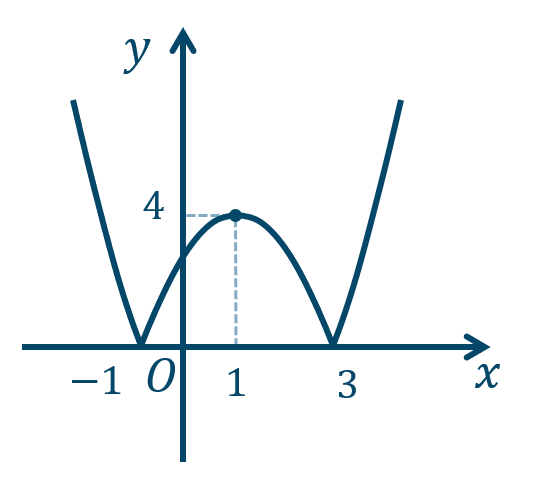
\({\small (3)}~\)
問題
p.121
7
\({\small (1)}~\)共有点をもつ
\(\left({\large \frac{-3+\sqrt{7}}{2}},0\right)~,~\left({\large \frac{-3-\sqrt{7}}{2}},0\right)\)
\({\small (2)}~\)共有点をもたない
\({\small (3)}~\)共有点をもつ
\(\left({\large \frac{3}{2}},0\right)\)
7
\({\small (1)}~\)共有点をもつ
\(\left({\large \frac{-3+\sqrt{7}}{2}},0\right)~,~\left({\large \frac{-3-\sqrt{7}}{2}},0\right)\)
\({\small (2)}~\)共有点をもたない
\({\small (3)}~\)共有点をもつ
\(\left({\large \frac{3}{2}},0\right)\)
p.121
8
\(\sqrt{5}\)
8
\(\sqrt{5}\)
p.121
9
\(m=-2\) のとき \((-1,0)\)
\(m=10\) のとき \((5,0)\)
9
\(m=-2\) のとき \((-1,0)\)
\(m=10\) のとき \((5,0)\)
p.121
10
\({\small (1)}~x<-{\large \frac{1}{3}}~,~{\large \frac{3}{2}}<x\)
\({\small (2)}~x≦1-\sqrt{10}~,~1+\sqrt{10}≦x\)
\({\small (3)}~{\large \frac{5-\sqrt{5}}{10}}<x<{\large \frac{5+\sqrt{5}}{10}}\)
10
\({\small (1)}~x<-{\large \frac{1}{3}}~,~{\large \frac{3}{2}}<x\)
\({\small (2)}~x≦1-\sqrt{10}~,~1+\sqrt{10}≦x\)
\({\small (3)}~{\large \frac{5-\sqrt{5}}{10}}<x<{\large \frac{5+\sqrt{5}}{10}}\)
p.121
11
\(m<-{\large \frac{3}{2}}~,~1<m\) のとき、2個
\(m=-{\large \frac{3}{2}}~,~1\) のとき、1個
\(-{\large \frac{3}{2}}<m<1\) のとき、0個
11
\(m<-{\large \frac{3}{2}}~,~1<m\) のとき、2個
\(m=-{\large \frac{3}{2}}~,~1\) のとき、1個
\(-{\large \frac{3}{2}}<m<1\) のとき、0個
p.121
12
\(5\) m以下
12
\(5\) m以下
p.121
13
\(-{\large \frac{1}{3}}≦m≦0\)
13
\(-{\large \frac{1}{3}}≦m≦0\)
p.121
14
\(m<{\large \frac{3-\sqrt{10}}{2}}\)
14
\(m<{\large \frac{3-\sqrt{10}}{2}}\)
演習問題 2次関数
演習問題A
p.122
1
\({\small (1)}~y=x^2-x+1\)
\({\small (2)}~y=2(x-3)^2-9\)
\(~,~y=2(x-5)^2-25\)
1
\({\small (1)}~y=x^2-x+1\)
\({\small (2)}~y=2(x-3)^2-9\)
\(~,~y=2(x-5)^2-25\)
p.122
2
\({\small (1)}~a>0~,~b<0~,~c<0\)
\(~,~b^2-4ac>0\)
\({\small (2)}~a>0~,~b>0~,~c>0\)
\(~,~b^2-4ac<0\)
\({\small (3)}~a<0~,~b>0~,~c<0\)
\(~,~b^2-4ac<0\)
\({\small (4)}~a<0~,~b<0~,~c<0\)
\(~,~b^2-4ac>0\)
2
\({\small (1)}~a>0~,~b<0~,~c<0\)
\(~,~b^2-4ac>0\)
\({\small (2)}~a>0~,~b>0~,~c>0\)
\(~,~b^2-4ac<0\)
\({\small (3)}~a<0~,~b>0~,~c<0\)
\(~,~b^2-4ac<0\)
\({\small (4)}~a<0~,~b<0~,~c<0\)
\(~,~b^2-4ac>0\)
p.122
3
\(y=x^2+2x-2\)
3
\(y=x^2+2x-2\)
p.122
4
\(a=2~,~b=6\)
または
\(a=-2~,~b=-2\)
4
\(a=2~,~b=6\)
または
\(a=-2~,~b=-2\)
p.122
5
\({\small (1)}~\)
\(1<a\) のとき
\(x=a\) で最小値 \(a^2-2a\)
\(0≦a≦1\) のとき
\(x=1\) で最小値 \(-1\)
\(a<0\) のとき
\(x=a+1\) で最小値 \(a^2-1\)
\({\small (2)}~\)
\(a<{\large \frac{1}{2}}\) のとき
\(x=a+1\) で最大値 \(a^2-1\)
\(a={\large \frac{1}{2}}\) のとき
\(x={\large \frac{1}{2}},{\large \frac{3}{2}}\) で最大値 \(-{\large \frac{3}{4}}\)
\({\large \frac{1}{2}}<a\) のとき
\(x=a\) で最大値 \(a^2-2a\)
5
\({\small (1)}~\)
\(1<a\) のとき
\(x=a\) で最小値 \(a^2-2a\)
\(0≦a≦1\) のとき
\(x=1\) で最小値 \(-1\)
\(a<0\) のとき
\(x=a+1\) で最小値 \(a^2-1\)
\({\small (2)}~\)
\(a<{\large \frac{1}{2}}\) のとき
\(x=a+1\) で最大値 \(a^2-1\)
\(a={\large \frac{1}{2}}\) のとき
\(x={\large \frac{1}{2}},{\large \frac{3}{2}}\) で最大値 \(-{\large \frac{3}{4}}\)
\({\large \frac{1}{2}}<a\) のとき
\(x=a\) で最大値 \(a^2-2a\)
p.122
6
\({\small (1)}~\)解なし
\({\small (2)}~a<1\) のとき、\(a<x<1\)
\(a>1\) のとき、\(1<x<a\)
\({\small (3)}~-1≦a<0~,~2<a≦3\)
6
\({\small (1)}~\)解なし
\({\small (2)}~a<1\) のとき、\(a<x<1\)
\(a>1\) のとき、\(1<x<a\)
\({\small (3)}~-1≦a<0~,~2<a≦3\)
演習問題B
p.123
7
\(a=-1-\sqrt{2}~,~{\large \frac{1}{2}}\)
7
\(a=-1-\sqrt{2}~,~{\large \frac{1}{2}}\)
p.123
8
\(0<a<2\) のとき
\(x=a\) で最大値 \(-a^2+4a\)
\(2≦a<2+2\sqrt{2}\) のとき
\(x=2\) で最大値 \(4\)
\(a=2+2\sqrt{2}\) のとき
\(x=2~,~2+2\sqrt{2}\) で最大値 \(4\)
\(2+2\sqrt{2}<a\) のとき
\(x=a\) で最大値 \(a^2-4a\)
8
\(0<a<2\) のとき
\(x=a\) で最大値 \(-a^2+4a\)
\(2≦a<2+2\sqrt{2}\) のとき
\(x=2\) で最大値 \(4\)
\(a=2+2\sqrt{2}\) のとき
\(x=2~,~2+2\sqrt{2}\) で最大値 \(4\)
\(2+2\sqrt{2}<a\) のとき
\(x=a\) で最大値 \(a^2-4a\)
p.123
9
\({\small (1)}~l=-2m^2+3m\)
\({\small (2)}~\)
\(m={\large \frac{3}{4}}\) のとき、最大値 \(l={\large \frac{9}{8}}\)
9
\({\small (1)}~l=-2m^2+3m\)
\({\small (2)}~\)
\(m={\large \frac{3}{4}}\) のとき、最大値 \(l={\large \frac{9}{8}}\)
p.123
10
\(m<-1~,~5<m\)
10
\(m<-1~,~5<m\)
p.123
11
\({\small (1)}~0<a≦{\large \frac{3}{2}}\)
\({\small (2)}~-{\large \frac{3}{2}}≦a<0~,~0<a≦4\)
\({\small (3)}~-{\large \frac{3}{2}}≦a<0~,~{\large \frac{3}{2}}<a≦4\)
11
\({\small (1)}~0<a≦{\large \frac{3}{2}}\)
\({\small (2)}~-{\large \frac{3}{2}}≦a<0~,~0<a≦4\)
\({\small (3)}~-{\large \frac{3}{2}}≦a<0~,~{\large \frac{3}{2}}<a≦4\)
p.123
12
\({\small (1)}~4<m<8\)
\({\small (2)}~m<-8\)
\({\small (3)}~m>8\)
12
\({\small (1)}~4<m<8\)
\({\small (2)}~m<-8\)
\({\small (3)}~m>8\)
p.123
13
\((0,0)~,~(2,8)\)
13
\((0,0)~,~(2,8)\)
次のページ「第4章 図形と計量」