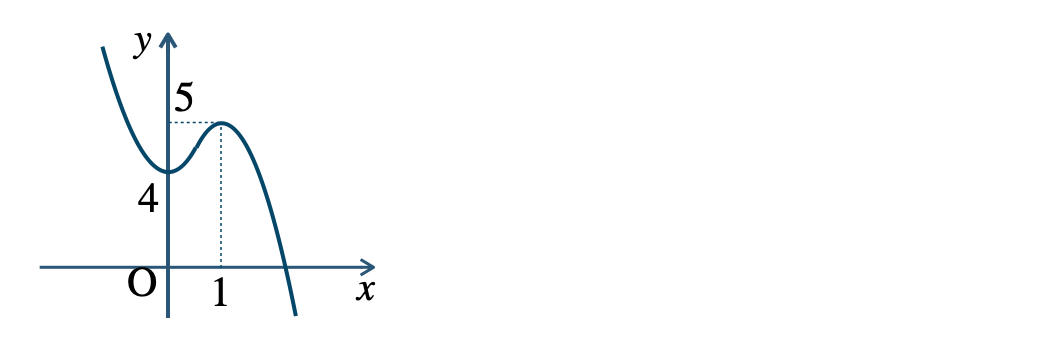東京書籍：Advanced数学Ⅱ

p.190 問1$$~~~7~{\rm m/s}$$

p.191 問2$${\small (1)}~3$$$${\small (2)}~19$$

p.191 問3$${\small (1)}~-2+h$$$${\small (2)}~2a-4+h$$→ 平均変化率

p.192 問4$${\small (1)}~5$$$${\small (2)}~-2$$→ 極限値

p.193 問5$$~~~6~{\rm m/s}$$

p.193 問6$${\small (1)}~4$$$${\small (2)}~-8$$$${\small (3)}~4a$$→ 微分係数

p.194 問7$$~~~-4$$

p.196 問8$$\begin{split}&f'(x)\\[2pt]~~=~&\lim_{h\to 0}{ \frac{\,(x+h)^2+7-(x^2+7)\,}{h}}\\[2pt]~~=~&\lim_{h\to 0}{ \frac{\,x^2+2xh+2h^2+7-x^2-7\,}{h}}\\[2pt]~~=~&\lim_{h\to 0}{ \frac{\,2xh+h^2\,}{h}}\\[2pt]~~=~&\lim_{h\to 0}(2x+h)\\[2pt]~~=~&2x\end{split}$$→ 導関数

p.197 問9$$~~~y’=4x^3$$

p.197 問10$$~~~y’=0$$

p.197 問11$$~~~y’=-12x^2$$

p.198 問12$$~~~y’=3x^2-1$$→ 微分の計算

p.198 問13[証明]
　(左辺)
\(=\{kf(x)+lg(x)\}’\)
\(=\{kf(x)\}’+\{lg(x)\}’\)
\(=kf'(x)+lg'(x)\)
したがって、
\(\{kf(x)+lg(x)\}’=kf'(x)+lg'(x)\)
[終]

p.199 問14$${\small (1)}~y’=-2$$$${\small (2)}~y’=-6x+1$$$${\small (3)}~y’=15x^2-8$$$${\small (4)}~y’=x^2-x-3$$$${\small (5)}~y’=-12x^2+12x+7$$

p.199 問15$${\small (1)}~y’=6x+14$$$${\small (2)}~y’=8x+12$$$${\small (3)}~y’=3x^2+4x+1$$$${\small (4)}~y’=3x^2-6x+3$$→ 微分の計算

p.200 問16$$~~~f'(2)=32~,~f'(-1)=11$$

p.200 問17$$~~~a=-2$$→ ２次関数の決定（微分係数の利用）

p.200 問18$${\small (1)}~{ \frac{\,dh\,}{dt}}=10-10t$$$${\small (2)}~{ \frac{\,dS\,}{dr}}=2\pi r$$

p.201 問題 9\({\small (1)}~\)[証明] \(y=a^2x^2+2abx+b^2\) より、微分すると、
　\(y’=2a^2x+2ab=2a(ax+b)\)
したがって、
\(y=(ax+b)^2\) ならば \(y’=2a(ax+b)\) [終]
 
\({\small (2)}~\)[証明]
\(y=a^3x^3+3a^2bx^2+3ab^2x+b^3\) より、微分すると、
　\(y’=3a^3x^2+6a^2bx+3a^2\)
　\(~~=3a(a^2x^2+2abx+b^2)\)
　\(~~=3a(ax+b)^2\)
したがって、
\(y=(ax+b)^3\) ならば \(y’=3a(ax+b)^2\) [終]

p.202 発展 問1$${\small (1)}~-7$$$${\small (2)}~5$$

p.203 発展 問2$${\small (1)}~39$$$${\small (2)}~1$$$${\small (3)}~4$$$${\small (4)}~-1$$

p.203 発展 問3[証明] 導関数の定義より、$$\small~~~~~~\left\{ f(x)+g(x) \right\}’$$$$\small~=\lim_{h\to 0}\frac{\,\{f(x+h)+g(x+h) \}-\{f(x)+g(x) \}\,}{\,h\,}$$$$\small~=\lim_{h\to 0}\frac{\,f(x+h)-f(x)+g(x+h)-g(x)\,}{\,h\,}$$$$\small~=\lim_{h\to 0}\frac{\,f(x+h)-f(x)\,}{\,h\,}+~\lim_{h\to 0}\frac{\,g(x+h)-g(x)\,}{\,h\,}$$$$\small~=f'(x)+g'(x)$$したがって、$$~~~\left\{ f(x)+g(x) \right\}’=f'(x)+g'(x)$$[終]

p.204 問1$$~~~y=3x-8$$

p.205 問2$$~~~y=-3x-5$$→ 接線の方程式①

p.205 問3$$~~~y=4x-3~,~y=-8x-15$$→ 接線の方程式②（外部の点から引いた接線）

p.207 問4\({\small (1)}~\)\(x≦0\) で減少
　　\(0≦x≦4\) で増加
　　\(x≧4\) で減少
\({\small (2)}~\)\(x≦-{\large \frac{1}{2}}\) で増加
　　\(-{\large \frac{1}{2}}≦x≦{\large \frac{1}{2}}\) で減少
　　\(x≧{\large \frac{1}{2}}\) で増加

p.209 問5\({\small (1)}~\)極大値なし
　　\(x=3\) で極小値 \(-4\)
\({\small (2)}~\)\(x=-1\) で極大値 \(5\)
　　\(x=3\) で極小値 \(-27\)

p.209 問6\({\small (1)}~\)

\({\small (2)}~\)

→ ３次関数のグラフと増減表

p.210 問7$$~~~f'(x)=3×2-6x+3=3(x-1)^2$$これより、\(f'(x)≧0\) となり、\(f(x)\) は常に増加する
したがって、\(f(x)\) は極値をもたない

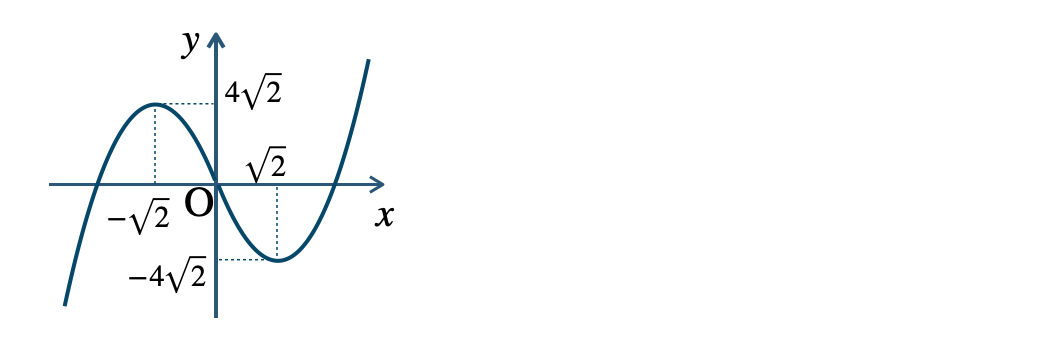
p.211 問8\({\small (1)}~\)\(x=-2\) で極小値 \(-{\large \frac{\,8\,}{3}}\)
　　\(x=0\) で極大値 \(0\)
　　\(x=1\) で極小値 \(-{\large \frac{5}{\,12\,}}\)

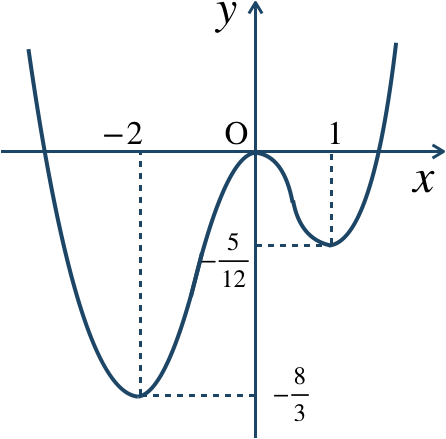
\({\small (2)}~\)極大値なし
　　\(x={\large \frac{\,3\,}{2}}\) で極小値 \(-{\large \frac{\,27\,}{16}}\)

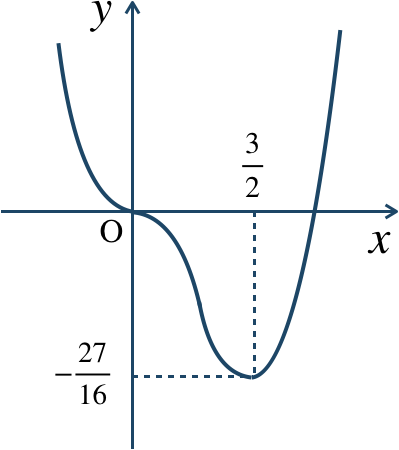
→ ４次関数のグラフと増減表

p.212 問9$$~~~a={ \frac{\,3\,}{2}}~,~b=6$$　\(x=2\) で極大値 \(10\)
　\(x=-1\) で極小値 \(-{\large \frac{7}{2}}\)
→ 極値の条件と関数の決定

p.213 問10\({\small (1)}~\)\(x=-1\) で最大値 \(3\)
　　\(x=-2\) で最小値 \(-4\)
\({\small (2)}~\)\(x=-2\) で最大値 \(43\)
　　\(x=-{\large \frac{1}{\,2\,}}\) で最小値 \(-{\large \frac{\,17\,}{4}}\)
→ ３次関数の最大値・最小値

p.214 問11　半径 \(20\) cm、高さ \(10\) cm

p.215 問12\({\small (1)}~\)３個
\({\small (2)}~\)２個
\({\small (3)}~\)１個
→ ３次方程式の解の個数①

p.216 問13　\(a<-1~,~0<a\) のとき、１個
　\(a=-1~,~0\) のとき、２個
　\(-1<a<0\) のとに、３個
→ ３次方程式の解の個数②（定数分離法）

p.217 問14[証明]
\(f(x)=(x^3+2)-3x\) とすると、
　\(f'(x)=3x^2-3=3(x+1)(x-1)\)
よって、\(x≧0\) での増減表は

\(x\)	\(0\)	\(\cdots\)	\(1\)	\(\cdots\)
\(f'(x)\)		\(-\)	\(0\)	\(+\)
\(f(x)\)	\(2\)	↘︎	\(0\)	↗︎

よって、\(x≧0\) で最小値が \(0\) であるので、
　\(f(x)≧0\)
したがって、\(x≧0\) のとき
　\(x^3+2≧3x\)
また、等号が成り立つときは \(x=1\) のとき [終]
→ ３次不等式の証明

p.218 問題 11$$~~~a=1$$

p.221 問1\(C\) は積分定数$$~~~{ \frac{1}{\,5\,}}x^5+C$$

p.221 問2\(C\) は積分定数$${\small (1)}~-2x+C$$$${\small (2)}~x^2-3x+C$$$${\small (3)}~3x^3-{ \frac{\,5\,}{2}}x^2-x+C$$

p.222 問3\(C\) は積分定数$${\small (1)}~3x^3-6x^2+4x+C$$$${\small (2)}~{ \frac{\,8\,}{3}}t^3+3t^2-9t+C$$→ 不定積分

p.223 問4$$~~~F(x)={ \frac{1}{\,3\,}}x^3+{ \frac{1}{\,2\,}}x^2-2x+1$$→ 不定積分と関数の決定

p.223 問5$$~~~f(x)=-2x^3+x^2$$→ 接線の傾きの条件と関数の決定

p.225 問6$${\small (1)}~{ \frac{2}{\,3\,}}$$$${\small (2)}~2$$$${\small (3)}~-14$$$${\small (4)}~{ \frac{\,31\,}{3}}$$

p.226 問7$${\small (1)}~{ \frac{\,21\,}{2}}$$$${\small (2)}~{ \frac{\,44\,}{3}}$$→ 定積分の計算

p.227 問8\({\small [4]}~\)[証明] \(f(x)\)の原始関数の１つを \(F(x)\) とすると、
　　\(\int_{a}^{a}f(x)dx\)
　\(=\left[ F(x) \right]_{a}^{a}\)
　\(=F(a)-F(a)=0\)
したがって、
　\(\int_{a}^{a}f(x)dx=0\) [終]
 
\({\small [5]}~\)[証明] \(f(x)\)の原始関数の１つを \(F(x)\) とすると、
　　\(\int_{b}^{a}f(x)dx\)
　\(=\left[ F(x) \right]_{b}^{a}\)
　\(=F(a)-F(b)\)
　\(=-\left(F(b)-F(a)\right)\)
　\(=-\left[ F(x) \right]_{a}^{b}\)
　\(=-\int_{a}^{b}f(x)dx\)
したがって、
　\(\int_{b}^{a}f(x)dx=-\int_{a}^{b}f(x)dx\) [終]

p.227 問9$${\small (1)}~0$$$${\small (2)}~18$$→ 定積分の計算

p.228 問10$$~~~f(x)=3x-4$$→ 定積分を含む式

p.229 問11$$~~~4x^2-x+2$$→ 定積分で表された関数

p.229 問12$${\small (1)}~f(x)=2x-3~,~a=-1~,~4$$$${\small (2)}~f(x)=3x^2+4~,~a=4$$→ 定積分で表された関数

p.232 問13$$~~~6$$

p.232 問14$${\small (1)}~{ \frac{\,4\,}{3}}$$$${\small (2)}~{ \frac{\,9\,}{2}}$$$${\small (3)}~{ \frac{2}{\,3\,}}$$→ 定積分と面積①（x軸と囲まれた面積）

p.233 問15$$~~~{ \frac{\,33\,}{2}}$$→ 定積分と面積③（区間付きの面積）

p.233 問16$$~~~8$$

p.235 問17$${\small (1)}~{ \frac{1}{\,6\,}}$$$${\small (2)}~{ \frac{\,9\,}{2}}$$$${\small (3)}~{ \frac{\,32\,}{3}}$$→ 定積分と面積②（２つの関数で囲まれた面積）

p.236 問18$$~~~{ \frac{\,22\,}{3}}$$→ 絶対値を含む関数の定積分

p.237 参考 問1$$~~~{ \frac{\,32\,}{3}}$$

p.238 問題 23$$~~~S_1=\int_{-1}^{0}(x^2+1-x^2)dx=1$$また、\(S_2\) は底辺 \(1\)、高さ \(1\) の平行四辺形であるので、$$~~~S_2=1\times 1=1$$したがって、面積 \(S_1\) と面積 \(S_2\) が等しい

p.239 参考 問1$${\small (1)}~y’=12(3x+5)^3$$$${\small (2)}~y’=-5(1-x)^4$$

p.239 参考 問2\(C\) は積分定数$$~~~{ \frac{1}{\,4\,}}(x-3)^4+C$$

p.239 参考 問3$$~~~{ \frac{\,11\,}{5}}$$

p.240 参考 問1$${\small (1)}~y=3x~,~y=-5x$$$${\small (2)}~{ \frac{\,4\,}{3}}$$

p.241 参考 問2$$~~~{ \frac{\,4\,}{3}}$$

p.245 練習問題Ｂ 14[証明] \(f(x)=px+q\) とすると、
　(左辺)
\(=\left\{{\Large [} {\large \frac{p}{2}}x^2+qx {\Large ]}_{0}^{1}\right\}^2\)
\(=\left({\large \frac{p}{2}}+q\right)^2\)
\(={\large \frac{p^2}{4}}+pq+q^2\)
　(右辺)
\(=\int_{0}^{1}(p^2x^2+2pqx+q^2)dx\)
\(={\Large [} {\large \frac{p^2}{3}}x^3+pqx^+q^2x {\Large ]}_{0}^{1}\)
\(={\large \frac{p^2}{3}}+pq+q^2\)
よって、
　(右辺)\(-\)(左辺)
\(=\left({\large \frac{p^2}{3}}+pq+q^2\right)\)
　　\(-\left({\large \frac{p^2}{4}}+pq+q^2\right)\)
\(={\large \frac{p^2}{12}}>0\)
したがって、
　\(\left\{\int_{0}^{1}(px+q)dx\right\}^2\)
　　　\(<\int_{0}^{1}(px+q)^2dx\)
[終]