東京書籍：Advanced数学Ⅰ

p.6
問1
\({\small (1)}~\)次数 \(4\)、係数 \(5\)
\({\small (2)}~\)次数 \(4\)、係数 \(1\)
\({\small (3)}~\)次数 \(0\)、係数 \(-7\)

p.6
問2
\({\small (1)}~\)次数 \(3\)、係数 \(4x^2\)
\({\small (2)}~\)次数 \(5\)、係数 \(-2a^2\)
→ 単項式の次数と係数

p.7
問3
\(-4x^2y+9xy-4\)

p.7
問4
\({\small (1)}~\)５次式、\(-8\)
\(x\) については３次式、\(y^4-8\)
\({\small (2)}~\)３次式、\(1\)
\(x\) については３次式、\(-y^2-4y+1\)

p.7
問5
\({\small (1)}~7x^3+5x^2-3x-2\)
\({\small (2)}~2x^2+(5y-1)x+(y^2+5y-4)\)
→ 多項式の次数と定数項

p.8
問6
\({\small (1)}~{\rm A+B}=4x^3-9x^2-x\)
\(~~~~~~{\rm A-B}=-2x^3+x^2+x-6\)
\({\small (2)}~{\rm A+B}=x^2-3xy+2y^2\)
\(~~~~~~{\rm A-B}=3x^2+3xy\)

p.8
問7
\({\small (1)}~11x-14\)
\({\small (2)}~7x^2+x+7\)
\({\small (3)}~11x^2-11x+27\)
→ 多項式の計算

p.9
問8
\({\small (1)}~a^8\)　\({\small (2)}~a^3b^9\)
\({\small (3)}~x^{17}\)　\({\small (4)}~x^{11}y^{14}\)

p.9
問9
\({\small (1)}~{\large \frac{1}{2}}a^7\)　\({\small (2)}~-4a^8b^5\)
\({\small (3)}~81x^{14}\)　\({\small (4)}~2x^8y^{11}\)
→ 累乗の計算

p.10
問10
\({\small (1)}~6x^2-21x\)
\({\small (2)}~15x^5-10x^4+5x^3\)
\({\small (3)}~-8x^3y+4x^2y^2-4xy^3\)

p.10
問11
\({\small (1)}~2x^2+15x+18\)
\({\small (2)}~15x^2+23x-28\)
\({\small (3)}~2x^3-27x+20\)
\({\small (4)}~8x^3-32x^2+20x-21\)
→ 分配法則と展開

p.11
問12
\({\small (1)}~9x^2+6xy+y^2\)
\({\small (2)}~64x^2-48xy+9y^2\)
\({\small (3)}~36x^2-25y^2\)
\({\small (4)}~x^2-5x-14\)

p.11
問13
\({\small (1)}~10x^2+9x+2\)
\({\small (2)}~6x^2+7x-20\)

p.12
問14
\({\small (1)}~4x^2-13xy+3y^2\)
\({\small (2)}~12x^2-5xy-2y^2\)
→ ２次式の展開と乗法公式

p.12
問15
\({\small (1)}~a^2+2ab+b^2-5a-5b\)
\({\small (2)}~a^2-2ab+b^2-4a+4b-21\)
\({\small (3)}~x^2-2xz+z^2-y^2\)
\({\small (4)}~x^2-y^2+2yz-z^2\)

p.12
問16
\({\small (1)}~a^2+b^2+c^2+2ab-2bc-2ca\)
\({\small (2)}~a^2+b^2+c^2-2ab+2bc-2ca\)
\({\small (3)}~x^2+4y^2+9z^2-4xy-12yz+6zx\)

p.12
問17
\({\small (1)}~x^4-29x^2+100\)
\({\small (2)}~x^4+10x^3+35x^2+50x+24\)
\({\small (3)}~a^4-8a^2b^2+16b^4\)
\({\small (4)}~16x^4-72x^2y^2+81y^4\)

p.13
問18
\(a^4-1\)
→ 式の展開の工夫

p.14
問19
\({\small (1)}~3a(3ab-2c)\)
\({\small (2)}~yz(3x^2+1)\)
\({\small (3)}~3a^2b^2(a-2b+4c)\)

p.14
問20
\({\small (1)}~(x+5y)(y-z)\)
\({\small (2)}~(4x+1)(y-2)\)
\({\small (3)}~(3a-b)(x-1)\)
\({\small (4)}~(a+2)(b-c)\)

p.15
問21
\({\small (1)}~(4x+1)^2\)
\({\small (2)}~(2x-7y)^2\)
\({\small (3)}~(8x+9y)(8x-9y)\)
\({\small (4)}~(x-2)(x+15)\)

p.15
問22
\({\small (1)}~x^2(5x+2y)(5x-2y)\)
\({\small (2)}~a(x+6)^2\)
\({\small (3)}~x(x+6)(x-8)\)
\({\small (4)}~(a-b)(x+y)(x-y)\)
→ ２次式の因数分解

p.16
問23
\({\small (1)}~(x+1)(2x+1)\)
\({\small (2)}~(x-2)(3x+1)\)
\({\small (3)}~(x+2)(5x-3)\)
\({\small (4)}~(2x-1)(4x+5)\)
\({\small (5)}~(2x-3)(3x+2)\)
\({\small (6)}~(2x-3)(2x-5)\)

p.17
問24
\({\small (1)}~(x+y)(7x+4y)\)
\({\small (2)}~(3x+2y)(4x-3y)\)
→ 因数分解（たすき掛け）
→ 【問題演習】因数分解（たすき掛け）

p.17
問25
\({\small (1)}~(a+5b)(a+3b)\)
\({\small (2)}~(3x+y-z)(3x-y+z)\)
\({\small (3)}~(x-y+9)(x-y-5)\)
\({\small (4)}~(2a+b-4)(2a+b-5)\)
→ 因数分解の工夫

p.18
問26
\({\small (1)}~(2y+1)(2y-1)(x-1)\)
\({\small (2)}~(a+3b)(a-3b)(a+c)\)
→ ２種類以上の文字を含む式の因数分解①（１次式）

p.18
問27
\({\small (1)}~(x+y+2)(x+2y+3)\)
\({\small (2)}~(x-2y+1)(2x+y-1)\)

p.19
問28
\((a+b)(b+c)(c+a)\)
→ 文字式のたすき掛け
→ ２種類以上の文字を含む式の因数分解②（２次式）

p.19
1
\(9x^2-32x+25\)

p.19
2
\(4x^3-2x^2+4~,~2x^3+4x^2-3x-8\)

p.19
3
\({\small (1)}~3x^3+20x^2-22x+5\)
\({\small (2)}~x^4-2x^3+3x^2-2x+1\)
\({\small (3)}~a^2-4b^2-{\large \frac{1}{4}}c^2-2bc\)
\({\small (4)}~x^4+6x^3-7x^2-36x+36\)

p.19
4
\({\small (1)}~2x(2x+1)(x-5)\)
\({\small (2)}~(2a+b)(4a-3b)\)
\({\small (3)}~(x-3)(x-4)\)
\({\small (4)}~-x(3x-2y)\)
\({\small (5)}~(2b-1)(2ab+a+1)\)
\({\small (6)}~(x+1)(x-a)\)
\({\small (7)}~(2x+y-1)(3x+2y+1)\)
\({\small (8)}~(a+b)(a-b)(a-c)\)

p.20
参考1
\({\small (1)}~(x+2)(x-2)(x+3)(x-3)\)
\({\small (2)}~(2x+1)(2x-1)(2x^2+3)\)

p.20
参考2
\({\small (1)}~(x^2+x+1)(x^2-x+1)\)
\({\small (2)}~(3x^2+x-1)(3x^2-x-1)\)
→ 複２次式の因数分解

p.21
発展1
\({\small (1)}~(a+b)^3\)
\(~=(a+b)(a+b)^2\)
\(~=(a+b)(a^2+2ab+b^2)\)
\(~=a^3+2a^2b+ab^2\)
\(~~~~~~~~+a^2b+2ab^2+b^3\)
\(~=a^3+3a^2b+3ab^2+b^3\)

\({\small (2)}~(a-b)^3\)
\(~=(a-b)(a-b)^2\)
\(~=(a-b)(a^2-2ab+b^2)\)
\(~=a^3-2a^2b+ab^2\)
\(~~~~~~~~-a^2b+2ab^2-b^3\)
\(~=a^3-3a^2b+3ab^2-b^3\)

p.21
発展2
\({\small (1)}~x^3+3x^2+3x+1\)
\({\small (2)}~8x^3-12x^2y+6xy^2-y^3\)
→ ３次式の展開

p.21
発展3
\({\small (1)}~(a+b)(a^2-ab+b^2)\)
\(~=a^3-a^2b+ab^2\)
\(~~~~~~~~+a^2b-ab^2+b^3\)
\(~=a^3+b^3\)

\({\small (2)}~(a-b)(a^2+ab+b^2)\)
\(~=a^3+a^2b+ab^2\)
\(~~~~~~~~-a^2b-ab^2-b^3\)
\(~=a^3-b^3\)

p.21
発展4
\({\small (1)}~(x+5)(x^2-5x+25)\)
\({\small (2)}~(4x-3y)(16x^2+12xy+9y^2)\)
→ ３次式の因数分解

p.22
問1
\({\small (1)}~{\large \frac{51}{25}}\)　\({\small (2)}~{\large \frac{5}{8}}\)

p.23
問2
\({\small (1)}~0.8\dot{3}\)　\({\small (2)}~0.\dot{1}\dot{8}\)
\({\small (3)}~0.1\dot{2}\dot{7}\)　\({\small (4)}~1.\dot{2}9\dot{7}\)

p.23
問3
\({\small (1)}~{\large \frac{4}{33}}\)　\({\small (2)}~{\large \frac{11}{90}}\)　\({\small (3)}~{\large \frac{137}{111}}\)
→ 循環小数と分数

p.23
問4
有理数 \({\large \frac{1}{7}}~,~0.2\dot{3}~,~\sqrt{25}\)
無理数 \(2\pi~,~\sqrt{7}\)

p.25
問5

p.25
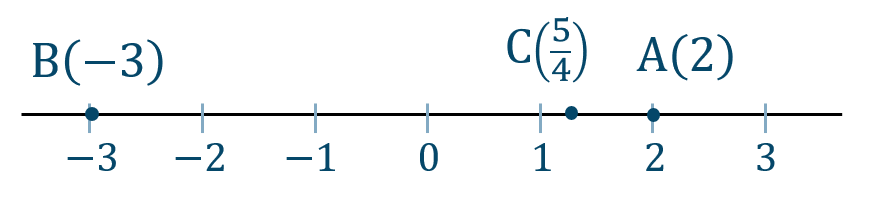
問6
\({\small (1)}~2.5\)　\({\small (2)}~{\large \frac{1}{3}}\)　\({\small (3)}~0\)

p.26
問7
\({\small (1)}~1\)　\({\small (2)}~{\large \frac{1}{12}}\)　\({\small (3)}~-1+\sqrt{3}\)
→ 絶対値の計算

p.26
問8
\({\small (1)}~3\)　\({\small (2)}~7\)　\({\small (3)}~6\)

p.26
問9
\(a=-2\) のとき、
　\(|-a|=|-(-2)|=|2|=2\)
　\(|a|=|-2|=2\)
したがって、\(|-a|=|a|\)

\(a=-2\) のとき、
　\(|a|^2=|-2|^2=4\)
　\(a^2=(-2)^2=4\)
したがって、\(|a|^2=a^2\)

p.26
問10
\(a=-3~,~b=2\) のとき、
　\(|ab|=|(-3)\cdot2|=|-6|=6\)
　\(|a||b|=|-3|\cdot|2|=3\cdot2=6\)
したがって、\(|ab|=|a||b|\)

\(a=-3~,~b=2\) のとき、
　\(\left|{\large \frac{a}{b}}\right|=\left|{\large \frac{-3}{2}}\right|={\large \frac{3}{2}}\)
　\({\large \frac{|a|}{|b|}}={\large \frac{|-3|}{|2|}}={\large \frac{3}{2}}\)
したがって、\(\left|{\large \frac{a}{b}}\right|={\large \frac{|a|}{|b|}}\)

p.27
問11
\({\small (1)}~\pm\sqrt{17}\)　\({\small (2)}~\pm5\)　\({\small (3)}~12\)

p.27
問12
\(-a+1\)

p.28
問13
\({\large \frac{\sqrt{a}}{\sqrt{b}}}\) を２乗すると、
　\(\left({\large \frac{\sqrt{a}}{\sqrt{b}}}\right)^2={\large \frac{a}{b}}\)
次に、\(\sqrt{{\large \frac{a}{b}}}\) を２乗すると、
　\(\left(\sqrt{{\large \frac{a}{b}}}\right)^2={\large \frac{a}{b}}\)
ここで、\({\large \frac{\sqrt{a}}{\sqrt{b}}}>0~,~\sqrt{{\large \frac{a}{b}}}>0\) より、
　\({\large \frac{\sqrt{a}}{\sqrt{b}}}=\sqrt{{\large \frac{a}{b}}}\)

p.28
問14
\({\small (1)}~2\sqrt{6}\)　\({\small (2)}~10\sqrt{17}\)
\({\small (3)}~-\sqrt{2}\)　\({\small (4)}~{\large \frac{5\sqrt{5}}{3}}+2\sqrt{2}\)

p.29
問15
\({\small (1)}~4\)　\({\small (2)}~16-4\sqrt{15}\)
→ 平方根の計算

p.29
問16
\({\small (1)}~{\large \frac{\sqrt{7}}{14}}\)　\({\small (2)}~{\large \frac{\sqrt{22}}{11}}\)　\({\small (3)}~{\large \frac{\sqrt{15}}{5}}\)

p.29
問17
\({\small (1)}~{\large \frac{\sqrt{6}-\sqrt{3}}{3}}\)　\({\small (2)}~3+\sqrt{6}\)　\({\small (3)}~{\large \frac{7+3\sqrt{5}}{2}}\)
→ 分母の有理化

p.30
問18
\({\small (1)}~\sqrt{7}\)　\({\small (2)}~{\large \frac{1}{2}}\)　\({\small (3)}~6\)
→ 対称式

p.30
発展1
\({\large \frac{11\sqrt{7}}{2}}\)

p.31
問19
整数部分 \(3\)、小数部分 \(\sqrt{10}-3\)

p.31
問20
整数部分 \(3\)、小数部分 \(\sqrt{5}-2\)
→ 整数部分と小数部分

p.32
5
\({\small (1)}~\pi\)　\({\small (2)}~\sqrt{2}-2\)

p.32
6
\({\small (1)}~-2\sqrt{3}\)　\({\small (2)}~2\sqrt{15}+2\sqrt{5}+2\sqrt{3}+9\)
\({\small (3)}~{\large \frac{8\sqrt{3}}{3}}\)　\({\small (4)}~2\sqrt{6}\)

p.32
7
\({\small (1)}~2\sqrt{2}\)　\({\small (2)}~{\large \frac{2+\sqrt{2}-\sqrt{6}}{4}}\)

p.32
8
\({\small (1)}~8\sqrt{5}\)　\({\small (2)}~18\)

p.32
9
\({\small (1)}~\)整数部分 \(5\)、小数部分 \(2\sqrt{7}-5\)
\({\small (2)}~\)整数部分 \(1\)、小数部分 \(2-\sqrt{2}\)

p.33
発展1
\({\small (1)}~\sqrt{3}+1\)　\({\small (2)}~2-\sqrt{2}\)
\({\small (3)}~\sqrt{6}+1\)　\({\small (4)}~2-\sqrt{3}\)
\({\small (5)}~\sqrt{7}+2\)　\({\small (6)}~{\large \frac{\sqrt{10}-\sqrt{2}}{2}}\)
→ 二重根号

p.34
問1
\(3a+4b≦500\)

p.35
問2
\({\small (1)}~a+3=-1~,~b+3=5\) より、
　\(a+3< b+3\) となる
次に、\(a-2=-6~,~b-2=0\) より、
　\(a-2< b-2\) となる
次に、\(2a=-8~,~2b=4\) より、
　\(2a< 2b\) となる
また、\({\large \frac{a}{2}}=-2~,~{\large \frac{b}{2}}=1\) より、
　\({\large \frac{a}{2}}<{\large \frac{b}{2}}\) となる

\({\small (2)}~a+3=-7~,~b+3=-3\) より、
　\(a+3< b+3\) となる
次に、\(a-2=-12~,~b-2=-8\) より、
　\(a-2< b-2\) となる
次に、\(2a=-20~,~2b=-12\) より、
　\(2a< 2b\) となる
また、\({\large \frac{a}{2}}=-5~,~{\large \frac{b}{2}}=-3\) より、
　\({\large \frac{a}{2}}<{\large \frac{b}{2}}\) となる

p.35
問3
\({\small (1)}~-2a=12~,~-2b=-8\) より、
　\(-2a> -2b\) となる
また、\({\large \frac{a}{-2}}=3~,~{\large \frac{b}{-2}}=-2\) より、
　\({\large \frac{a}{-2}}>{\large \frac{b}{-2}}\) となる

\({\small (2)}~-2a=16~,~-2b=8\) より、
　\(-2a> -2b\) となる
また、\({\large \frac{a}{-2}}=4~,~{\large \frac{b}{-2}}=2\) より、
　\({\large \frac{a}{-2}}>{\large \frac{b}{-2}}\) となる

p.36
問4
\({\small (1)}~x>3\)　\({\small (2)}~x≦4\)

p.37
問5
\({\small (1)}~x<2\)　\({\small (2)}~x≧{\large \frac{5}{2}}\)

p.37
問6
\({\small (1)}~x<2\)　\({\small (2)}~x≧-3\)
\({\small (3)}~x<3\)　\({\small (4)}~x≧{\large \frac{14}{3}}\)
→ １次不等式の解

p.38
問7
\({\small (1)}~-3< x < 1\)　\({\small (2)}~2≦ x < 4\)
\({\small (3)}~-{\large \frac{1}{3}}≦ x ≦3\)

p.39
問8
\({\small (1)}~x< -1\)　\({\small (2)}~x≦ 4\)

p.39
問9
\(-15≦x≦-3\)
→ 連立不等式の解

p.40
問10
\(900\)
→ 不等式の文章問題

p.41
問11
\({\small (1)}~x=6~,~-2\)　\({\small (2)}~x=-3~,~-11\)
\({\small (3)}~x=2~,~3\)

p.41
問12
\({\small (1)}~-2< x < 2\)　\({\small (2)}~-7≦x≦3\)
\({\small (3)}~x< 1~,~4< x\)
→ 絶対値を含む方程式と不等式

p.42
参考1
\({\small (1)}~x=1~,~5\)　\({\small (2)}~x=2\)

p.43
参考2
\({\small (1)}~1≦x≦5\)　\({\small (2)}~x< 1~,~4< x\)
\({\small (3)}~x< -1\)
→ 場合分けの必要な絶対値を含む方程式と不等式

p.44
10
\({\small (1)}~x< -3\)　\({\small (2)}~x≦-{\large \frac{9}{2}}\)

p.44
11
\({\small (1)}~4≦x≦{\large \frac{20}{3}}\)　\({\small (2)}~x≧-{\large \frac{1}{2}}\)

p.44
12
\({\small (1)}~x=-3~,~{\large \frac{1}{3}}\)　\({\small (2)}~-{\large \frac{5}{2}}< x< -1\)
\({\small (3)}~x≦-1~,~{\large \frac{9}{5}}≦x\)

p.44
13
\(-5,-4,-3,-2,-1,0,1,2\)
→ 不等式を満たす整数の解

p.44
14
ばら \(7\) 本、ゆり \(8\) 本

p.44
15
りんご \(15\) 個、かき \(8\) 個

p.45
発展1
①、③

p.45
発展2
① \(s^2-2t\)　② \(s^2-4t\)

p.45
発展3
②、③

p.46
1
\({\small (1)}~x^2+5xy+8y^2\)
\({\small (2)}~3x^3y+6x^2y^2-3xy^3\)

p.46
2
\({\small (1)}~a^2-b^2-c^2+d^2+2ad+2bc\)
\({\small (2)}~x^8-256\)

p.46
3
\({\small (1)}~(x-y+z)(x-y-z)\)
\({\small (2)}~(x-y+2)(2x-y+3)\)
\({\small (3)}~(x+y)(x-y)(y-z)\)
\({\small (4)}~(x+3)(x-2)(x^2+x-8)\)

p.46
4
\(-1+\sqrt{5}\)

p.46
5
\({\small (1)}~8\)　\({\small (2)}~62\)

p.46
6
\({\small (1)}~-5< x≦{\large \frac{10}{3}}\)　\({\small (2)}~x> 11\)
\({\small (3)}~x=3\)

p.46
7
\(21\) 本

p.47
8
\(2\)

p.47
9
\({\small (1)}~(a-b)(b-c)(c-a)\)
\({\small (2)}~(ax-a-b)(bx-a+b)\)
\({\small (3)}~(a+b+1)(a+c+1)\)
\({\small (4)}~(a+b)(b+c)(c+a)\)

p.47
10
\({\small (1)}~a=2~,~b={\large \frac{\sqrt{7}-1}{2}}\)　\({\small (2)}~10\)

p.47
11
\(2b\)

p.47
12
\(3≦a < 4\)

p.47
13
\({\small (1)}~k> 2\)　\({\small (2)}~k≧ 6\)