文字数が多く、重くなるのでページを分割しています。
各章は下のリンクまたはページ下の「次へ」をクリックしてください。
第1章 式と証明
第2章 複素数と方程式
第3章 図形と方程式
第4章 三角関数
第5章 指数関数と対数関数
第6章 微分法と積分法
第5章 指数関数と対数関数
第1節 指数関数
p.164 練習1$${\small (1)}~1$$$${\small (2)}~{ \frac{1}{\,9\,}}$$$${\small (3)}~{ \frac{1}{\,1000\,}}$$$${\small (5)}~-{ \frac{1}{\,125\,}}$$$${\small (6)}~25$$
p.165 問1$${\small (1)}~a$$$${\small (2)}~a^6$$$${\small (3)}~{ \frac{b^2}{\,a^2\,}}$$$${\small (4)}~{ \frac{1}{\,a^3\,}}$$
p.165 練習2$${\small (1)}~{ \frac{1}{\,a^2\,}}$$$${\small (2)}~a^3$$$${\small (3)}~{ \frac{\,a^3\,}{b^3}}$$$${\small (4)}~{ \frac{1}{\,a^2\,}}$$→ 指数法則の基本
p.166 練習3$${\small (1)}~7$$$${\small (2)}~2$$$${\small (3)}~0.1$$
p.167 問2[証明]
性質2$$~\left(\frac{\,\sqrt[\large n]{a}\,}{\sqrt[\large n]{b}}\right)^n=\frac{\,(\sqrt[\large n]{a})^n\,}{(\sqrt[\large n]{a})^n}=\frac{\,a\,}{b}$$\({\large \frac{\,\sqrt[\large n]{a}\,}{\sqrt[\large n]{b}}}>0\) より、両辺に \(n\) 乗根をとると、$$~~~\frac{\,\sqrt[\large n]{a}\,}{\sqrt[\large n]{b}}=\sqrt[\large n]{\frac{\,a\,}{b}}$$
性質3$$~\{(\sqrt[\large n]{a})^m\}^n=(\sqrt[\large n]{a})^{mn}$$$$~~~~~~=\{(\sqrt[\large n]{a})^n\}^m=a^m$$\((\sqrt[\large n]{a})^m>0\) より、両辺に \(n\) 乗根をとると、$$~~~(\sqrt[\large n]{a})^m=\sqrt[\large n]{a^m}$$
性質4$$~\left(\sqrt[\large m]{\sqrt[\large n]{a}}\right)^{mn}=\left\{\left(\sqrt[\large m]{\sqrt[\large n]{a}}\right)^{m}\right\}^n$$$$~~~~~~=(\sqrt[\large n]{a})^n=a$$\(\sqrt[\large m]{\sqrt[\large n]{a}}>0\) より、両辺に \(mn\) 乗根をとると、$$~~~\sqrt[\large m]{\sqrt[\large n]{a}}=\sqrt[\large mn]{a}$$
性質5$$~(\sqrt[\large n]{a^m})^{np}=\left\{ \left(\sqrt[\large n]{a^m} \right)^{n} \right\}^p$$$$~~~~~~=(a^m)^p=a^{mp}$$\(\sqrt[\large n]{a^m}>0\) より、両辺に \(n\) 乗根をとると、$$~~~\sqrt[\large n]{a^m}=\sqrt[\large np]{a^{mp}}$$[終]
性質2$$~\left(\frac{\,\sqrt[\large n]{a}\,}{\sqrt[\large n]{b}}\right)^n=\frac{\,(\sqrt[\large n]{a})^n\,}{(\sqrt[\large n]{a})^n}=\frac{\,a\,}{b}$$\({\large \frac{\,\sqrt[\large n]{a}\,}{\sqrt[\large n]{b}}}>0\) より、両辺に \(n\) 乗根をとると、$$~~~\frac{\,\sqrt[\large n]{a}\,}{\sqrt[\large n]{b}}=\sqrt[\large n]{\frac{\,a\,}{b}}$$
性質3$$~\{(\sqrt[\large n]{a})^m\}^n=(\sqrt[\large n]{a})^{mn}$$$$~~~~~~=\{(\sqrt[\large n]{a})^n\}^m=a^m$$\((\sqrt[\large n]{a})^m>0\) より、両辺に \(n\) 乗根をとると、$$~~~(\sqrt[\large n]{a})^m=\sqrt[\large n]{a^m}$$
性質4$$~\left(\sqrt[\large m]{\sqrt[\large n]{a}}\right)^{mn}=\left\{\left(\sqrt[\large m]{\sqrt[\large n]{a}}\right)^{m}\right\}^n$$$$~~~~~~=(\sqrt[\large n]{a})^n=a$$\(\sqrt[\large m]{\sqrt[\large n]{a}}>0\) より、両辺に \(mn\) 乗根をとると、$$~~~\sqrt[\large m]{\sqrt[\large n]{a}}=\sqrt[\large mn]{a}$$
性質5$$~(\sqrt[\large n]{a^m})^{np}=\left\{ \left(\sqrt[\large n]{a^m} \right)^{n} \right\}^p$$$$~~~~~~=(a^m)^p=a^{mp}$$\(\sqrt[\large n]{a^m}>0\) より、両辺に \(n\) 乗根をとると、$$~~~\sqrt[\large n]{a^m}=\sqrt[\large np]{a^{mp}}$$[終]
p.167 練習4$${\small (1)}~3$$$${\small (2)}~2$$$${\small (3)}~5$$$${\small (4)}~3$$$${\small (5)}~\sqrt{2}$$→ 累乗根
p.168 練習5$${\small (1)}~2$$$${\small (2)}~25$$$${\small (3)}~{ \frac{1}{\,125\,}}$$→ 指数法則の拡張
p.169 練習6$${\small (1)}~2$$$${\small (2)}~{ \frac{3}{\,4\,}}$$$${\small (3)}~3$$$${\small (4)}~a\sqrt[\large 3]{a^2}$$$${\small (5)}~a$$→ 指数法則を用いた計算
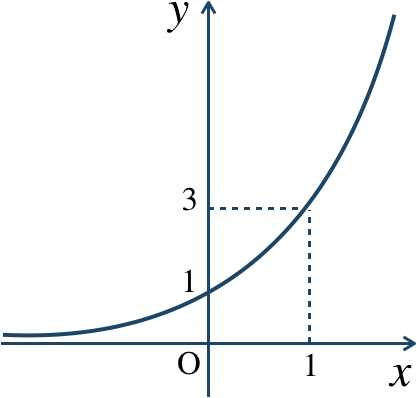
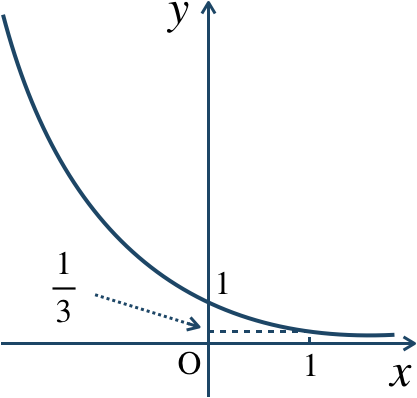
p.170 問3[証明] \(f(x)=2^x\) とすると、$$~~~f(-x)=2^{-x}=\left(\frac{1}{\,2\,}\right)^x$$これより、\(y\) 軸に関して対称である [終]

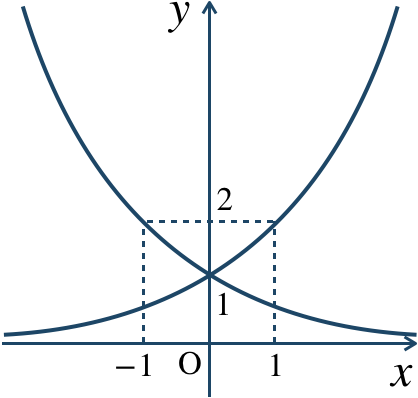
p.171 深める\(x<0\) のとき、\(y=2^x\) のグラフが \(y=3^x\) のグラフより上にくる
\(x>0\) のとき、\(y=3^x\) のグラフが \(y=2^x\) のグラフより上にくる
\(x>0\) のとき、\(y=3^x\) のグラフが \(y=2^x\) のグラフより上にくる
p.172 練習8$${\small (1)}~\sqrt[\large 3]{3}<\sqrt[\large 7]{27}<\sqrt[\large 4]{9}$$$${\small (2)}~\sqrt[\large 4]{{ \frac{1}{\,8\,}}}<\sqrt[\large 3]{{ \frac{1}{\,4\,}}}<\sqrt{{ \frac{1}{\,2\,}}}$$
→ 指数の大小比較
→ 指数の大小比較
p.173 練習9$${\small (1)}~x={ \frac{2}{\,3\,}}$$$${\small (2)}~x=3$$$${\small (3)}~x=1$$$${\small (4)}~x<5$$$${\small (5)}~x≧5$$$${\small (6)}~x>{ \frac{1}{\,2\,}}$$→ 指数方程式
→ 指数不等式
→ 指数不等式
p.173 練習10$${\small (1)}~x=2$$$${\small (2)}~x=-1~,~1$$$${\small (3)}~x>3$$$${\small (4)}~x>1$$→ 指数関数を含む2次方程式
→ 指数関数を含む2次不等式
→ 指数関数を含む2次不等式
p.174 問題4\({\small (1)}~\)



\({\small (2)}~\)
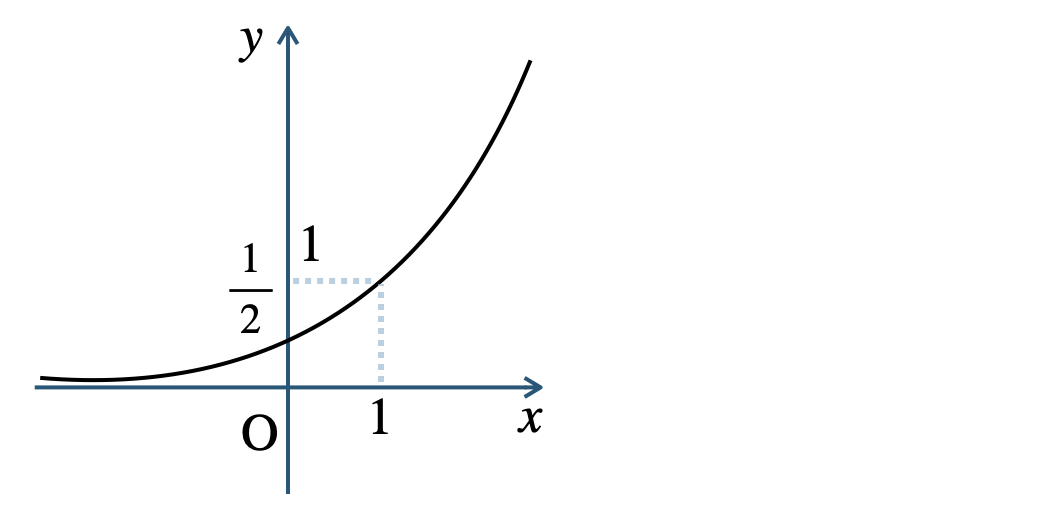
第2節 対数関数
p.175 練習11$${\small (1)}~5=\log_{3}243$$$${\small (2)}~{ \frac{1}{\,3\,}}=\log_{8}2$$$${\small (3)}~-1=\log_{10}0.1$$
p.175 練習12$${\small (1)}~2^3=8$$$${\small (2)}~10^{-5}={ \frac{1}{\,100000\,}}$$$${\small (3)}~3^{{ \frac{1}{\,2\,}}}=\sqrt{3}$$→ 指数と対数
p.176 練習13$${\small (1)}~5$$$${\small (2)}~{ \frac{\,3\,}{2}}$$$${\small (3)}~-3$$$${\small (4)}~{ \frac{1}{\,2\,}}$$$${\small (5)}~-2$$$${\small (6)}~-3$$→ 対数の値
p.177 練習14$${\small (1)}~2$$$${\small (2)}~-1$$$${\small (3)}~{ \frac{1}{\,3\,}}$$$${\small (4)}~{ \frac{\,3\,}{2}}$$→ 対数の計算
p.178 練習15$${\small (1)}~{ \frac{\,3\,}{2}}$$$${\small (2)}~{ \frac{1}{\,3\,}}$$$${\small (3)}~3$$→ 底の変換公式
p.178 問4[証明] 底の変換公式より、$$~~~~~~\log_{a}b \cdot\log_{b}c\cdot\log_{c}a$$$$~=\log_{a}b \cdot\frac{\,\log_{a}c\,}{\log_{a}b}\cdot\frac{\,\log_{a}a\,}{\log_{a}c}$$$$~=\log_{a}a=1$$[終]
p.178 練習16[証明] 底の変換公式より、$$~~~~~~\log_{a}b \cdot\log_{b}c\cdot\log_{c}d$$$$~=\log_{a}b \cdot\frac{\,\log_{a}c\,}{\log_{a}b}\cdot\frac{\,\log_{a}d\,}{\log_{a}c}$$$$~=\log_{a}d$$[終]
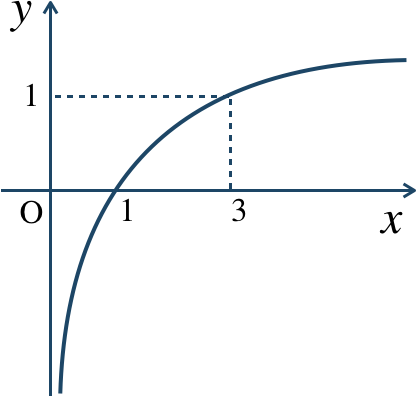
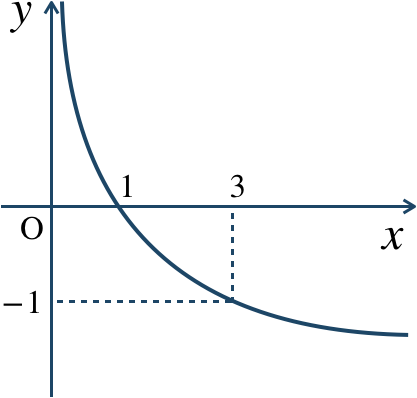
p.180 問5[証明] \(f(x)=\log_{2}x\) とすると、底の変換公式より、$$~\log_{{\large \frac{1}{\,2\,}}}x=\frac{\,\log_{2}x\,}{\log_{2}{\large \frac{1}{\,2\,}}}$$$$~~~~~~=-\log_{2}x=-f(x)$$したがって、\(x\) 軸に関して対称である [終]


p.181 練習18$$~~~{ \frac{1}{\,2\,}}<\log_{3}2<\log_{9}6$$→ 対数の大小比較
p.181 練習19$${\small (1)}~x=3\sqrt{3}$$$${\small (2)}~x=8$$$${\small (3)}~0<x≦0.25$$
p.181 練習20$${\small (1)}~x=18$$$${\small (2)}~-2<x<7$$$${\small (3)}~x≧{ \frac{\,10\,}{9}}$$→ 対数方程式
→ 対数不等式
→ 対数不等式
p.182 練習21$${\small (1)}~x=9$$$${\small (2)}~x=3$$
p.182 深める解が \(x=-1~,~8\) となり、同じにはならない
p.184 問6$${\small (1)}~1.8156$$$${\small (2)}~3.4742$$$${\small (3)}~-0.0410$$
p.184 練習24$${\small (1)}~1.2552$$$${\small (2)}~-0.2219$$$${\small (3)}~1.3980$$$${\small (4)}~3.0960$$
p.185 練習25 \(31\) 桁
p.186 問7$$~~~n=10$$
p.186 練習27$$~~~n=25$$
p.186 練習28 \(7\) 枚
p.187 研究 練習1\({\small (1)}~\)[証明]
\(\log_{2}5>0\) であり、これが有理数であると仮定すると、自然数 \(m~,~n\) を用いて$$~~~\log_{2}5=\frac{\,m\,}{n}$$これより、$$~~~2^{\large \frac{\,m\,}{n}}=5~\Leftrightarrow~2^m=5^n$$となるが、左辺が2の倍数であるが右辺は2の倍数でないので矛盾する
したがって、\(\log_{2}5\) は無理数である [終]
\({\small (2)}~\)[証明]$$~~~~~~\log_{2}10$$$$~=\log_{2}(2\times5)=1+\log_{2}5$$(1)より、\(\log_{2}5\) は無理数であるので、\(1+\log_{2}5\) も無理数である
したがって、\(\log_{2}10\) は無理数である [終]
\({\small (3)}~\)[証明] 底の変換公式より、$$~~~\log_{10}2=\frac{1}{\,\log_{2}10\,}$$(2)より、\(\log_{2}10\) は無理数であるので、\({\large \frac{1}{\,\log_{2}10\,}}\) も無理数である
したがって、\(\log_{10}2\) は無理数である [終]
\(\log_{2}5>0\) であり、これが有理数であると仮定すると、自然数 \(m~,~n\) を用いて$$~~~\log_{2}5=\frac{\,m\,}{n}$$これより、$$~~~2^{\large \frac{\,m\,}{n}}=5~\Leftrightarrow~2^m=5^n$$となるが、左辺が2の倍数であるが右辺は2の倍数でないので矛盾する
したがって、\(\log_{2}5\) は無理数である [終]
\({\small (2)}~\)[証明]$$~~~~~~\log_{2}10$$$$~=\log_{2}(2\times5)=1+\log_{2}5$$(1)より、\(\log_{2}5\) は無理数であるので、\(1+\log_{2}5\) も無理数である
したがって、\(\log_{2}10\) は無理数である [終]
\({\small (3)}~\)[証明] 底の変換公式より、$$~~~\log_{10}2=\frac{1}{\,\log_{2}10\,}$$(2)より、\(\log_{2}10\) は無理数であるので、\({\large \frac{1}{\,\log_{2}10\,}}\) も無理数である
したがって、\(\log_{10}2\) は無理数である [終]
p.188 問題5
\(x\) 軸に関して対称
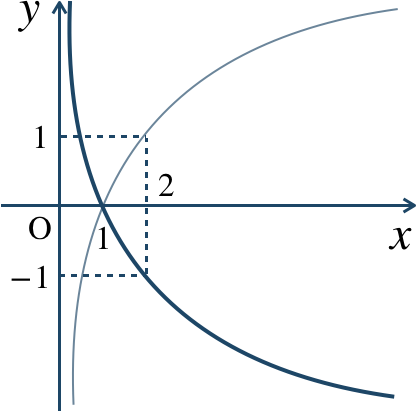
\(x\) 軸に関して対称
演習問題 指数関数と対数関数
p.189 演習問題A 2\({\small (1)}~\)

\({\small (2)}~\)

\({\small (3)}~\)

\({\small (2)}~\)
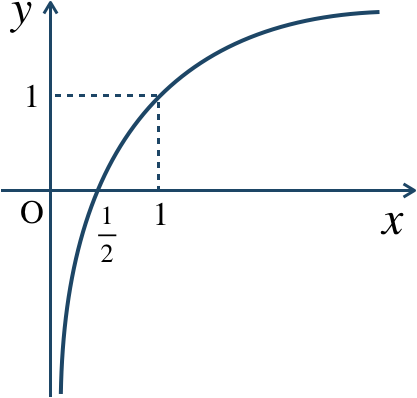
\({\small (3)}~\)
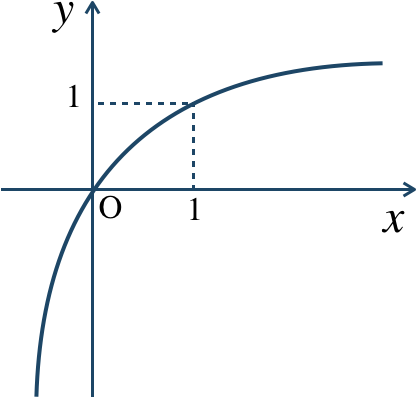
p.189 演習問題B 10[証明] 常用対数をとると、$$~~~\log_{10}2^x=\log_{10}3^y=\log_{10}6^z$$よって、$$~~~x\log_{10}2=y\log_{10}3=z\log_{10}6$$これより、$$~~~x=\frac{\,z\log_{10}6\,}{\log_{10}2}~,~y=\frac{\,z\log_{10}6\,}{\log_{10}3}$$ここで、
(左辺)$$~=\frac{1}{\,x\,}+\frac{1}{\,y\,}$$$$~=\frac{\,\log_{10}2\,}{z\log_{10}6}+\frac{\,\log_{10}3\,}{z\log_{10}6}$$$$~=\frac{\,\log_{10}2+\log_{10}3\,}{z\log_{10}6}$$$$~=\frac{\log_{10}6}{\,z\log_{10}6\,}=\frac{1}{\,z\,}$$したがって、$$~~~\frac{1}{\,x\,}+\frac{1}{\,y\,}=\frac{1}{\,z\,}$$[終]
(左辺)$$~=\frac{1}{\,x\,}+\frac{1}{\,y\,}$$$$~=\frac{\,\log_{10}2\,}{z\log_{10}6}+\frac{\,\log_{10}3\,}{z\log_{10}6}$$$$~=\frac{\,\log_{10}2+\log_{10}3\,}{z\log_{10}6}$$$$~=\frac{\log_{10}6}{\,z\log_{10}6\,}=\frac{1}{\,z\,}$$したがって、$$~~~\frac{1}{\,x\,}+\frac{1}{\,y\,}=\frac{1}{\,z\,}$$[終]
p.189 演習問題B 11\({\small (1)}~126\)桁
\({\small (2)}~\)[証明] 常用対数をとると、
\(\log_{10}10^{0.52}=0.52\)
\(\log_{10}4=\log_{10}2^2\)
\(=2\log_{10}2=0.602\)
よって、
\(\log_{10}10^{0.52}<\log_{10}4\)
したがって、
\(10^{0.52<4}\) [終]
\({\small (3)}~3\)
\({\small (2)}~\)[証明] 常用対数をとると、
\(\log_{10}10^{0.52}=0.52\)
\(\log_{10}4=\log_{10}2^2\)
\(=2\log_{10}2=0.602\)
よって、
\(\log_{10}10^{0.52}<\log_{10}4\)
したがって、
\(10^{0.52<4}\) [終]
\({\small (3)}~3\)
次のページ「第6章 微分法と積分法」